When a circle rolls on the inside of a fixed circle, any point P on the circumference
Question:
When a circle rolls on the inside of a fixed circle, any point P on the circumference of the rolling circle describes a hypocycloid. Let the fixed circle be x2 + y2 = a2, let the radius of the rolling circle be b, and let the initial position of the tracing point P be A(a, 0). Find parametric equations for the hypocycloid, using as the parameter the angle θ from the positive x-axis to the line joining the circles’ centers. In particular, if b = a/4, as in the accompanying figure, show that the hypocycloid is the astroid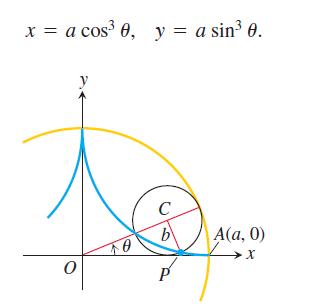
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

Thomas Calculus Early Transcendentals
ISBN: 9780321884077
13th Edition
Authors: Joel R Hass, Christopher E Heil, Maurice D Weir
Question Posted:





