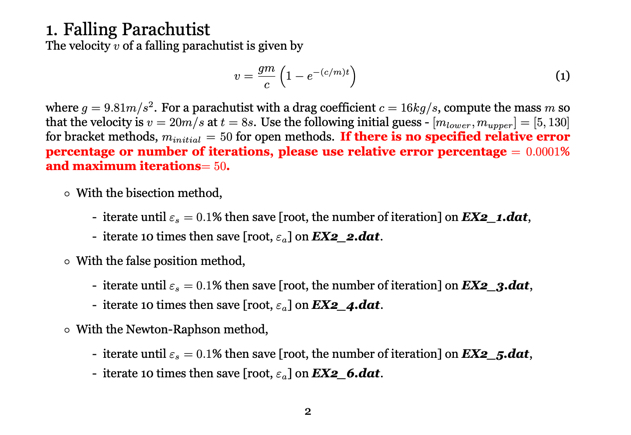

1. Falling Parachutist The velocity v of a falling parachutist is given by v=g (1-e-e/m)) (1) where g = 9.81m/s2. For a parachutist with a drag coefficient c=16kg/s, compute the mass m so that the velocity is v = 20m/s at t = 8s. Use the following initial guess - [mtower, Mupper) = (5, 130] for bracket methods, Minitial = 50 for open methods. If there is no specified relative error percentage or number of iterations, please use relative error percentage = 0.0001% and maximum iterations= 50. o With the bisection method, - iterate until , = 0.1% then save [root, the number of iteration) on EX2_1.dat, - iterate 10 times then save [root, ea] on EX2_2.dat. . With the false position method, - iterate until , = 0.1% then save [root, the number of iteration) on EX2_3.dat, - iterate 10 times then save [root, ea] on EX2_4.dat. . With the Newton-Raphson method, - iterate until , = 0.1% then save [root, the number of iteration) on EX2_5.dat, - iterate 10 times then save [root, ea) on EX2_6.dat. . With the Secant method, - with Minitial1 = 50 and minitial2 = 55, iterate until , = 0.1% then save [root, the number of iteration) on EX2_7.dat, - with Minitiall = 50 and Minitial2 = 55, iterate 10 times then save [root, Jon EX2_8.dat. o With the Modified Secant method, - with 8 = 0.1, iterate untile, = 0.1% then save [root, the number of iteration]on EX2_9.dat, - with 8 = 0.1, iterate 10 times then save [root, ea) on EX2_10.dat. . With the MixBisec False method, - with (Mlower, Mupper) = [5, 130) , iterate until = 0.1% then save [root, the number of iteration on EX2_11.dat, - with (mlower, Mupper- [5,130), iterate 10 times then save [root, Jon EX2_12.dat, - with (mlower, Mupper) = [5, 130), iterate until , = 0.01% then save [root, the number of iteration] on EX2_13.dat, - with (Mlower, Mupper] = [5, 130] , iterate 20 times then save [root, al on EX2_14.dat. 1. Falling Parachutist The velocity v of a falling parachutist is given by v=g (1-e-e/m)) (1) where g = 9.81m/s2. For a parachutist with a drag coefficient c=16kg/s, compute the mass m so that the velocity is v = 20m/s at t = 8s. Use the following initial guess - [mtower, Mupper) = (5, 130] for bracket methods, Minitial = 50 for open methods. If there is no specified relative error percentage or number of iterations, please use relative error percentage = 0.0001% and maximum iterations= 50. o With the bisection method, - iterate until , = 0.1% then save [root, the number of iteration) on EX2_1.dat, - iterate 10 times then save [root, ea] on EX2_2.dat. . With the false position method, - iterate until , = 0.1% then save [root, the number of iteration) on EX2_3.dat, - iterate 10 times then save [root, ea] on EX2_4.dat. . With the Newton-Raphson method, - iterate until , = 0.1% then save [root, the number of iteration) on EX2_5.dat, - iterate 10 times then save [root, ea) on EX2_6.dat. . With the Secant method, - with Minitial1 = 50 and minitial2 = 55, iterate until , = 0.1% then save [root, the number of iteration) on EX2_7.dat, - with Minitiall = 50 and Minitial2 = 55, iterate 10 times then save [root, Jon EX2_8.dat. o With the Modified Secant method, - with 8 = 0.1, iterate untile, = 0.1% then save [root, the number of iteration]on EX2_9.dat, - with 8 = 0.1, iterate 10 times then save [root, ea) on EX2_10.dat. . With the MixBisec False method, - with (Mlower, Mupper) = [5, 130) , iterate until = 0.1% then save [root, the number of iteration on EX2_11.dat, - with (mlower, Mupper- [5,130), iterate 10 times then save [root, Jon EX2_12.dat, - with (mlower, Mupper) = [5, 130), iterate until , = 0.01% then save [root, the number of iteration] on EX2_13.dat, - with (Mlower, Mupper] = [5, 130] , iterate 20 times then save [root, al on EX2_14.dat








