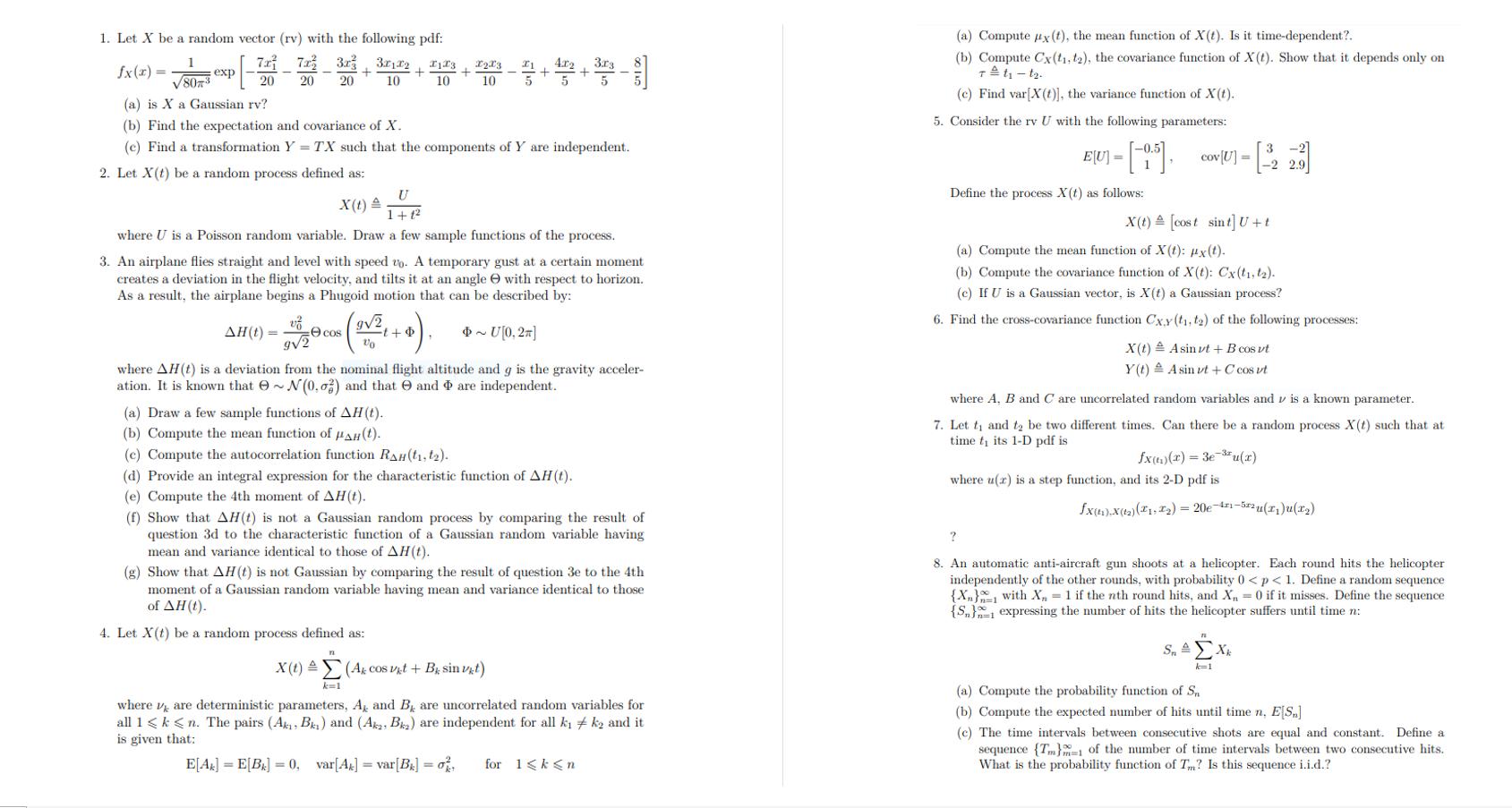
1. Let X be a random vector (rv) with the following pdf: 7x7x 3x3 fx(x) = (a) is X a Gaussian rv? (b) Find the expectation and covariance of X. (c) Find a transformation Y = TX such that the components of Y are independent. 2. Let X(t) be a random process defined as: X(t) = where U is a Poisson random variable. Draw a few sample functions of the process. 3. An airplane flies straight and level with speed to. A temporary gust at a certain moment creates a deviation in the flight velocity, and tilts it at an angle with respect to horizon. As a result, the airplane begins a Phugoid motion that can be described by: $~ U[0, 2] 92 from the nominal flight altitude and g is the gravity acceler- ~ N(0.02) and that and are independent. 1 803 : exp ------ + + 10 20 20 20 AH(t) = where AH(t) is a deviation ation. It is known that cos (92 gv Vo 10 U 1+1 TL t + $ k=1 10 (a) Draw a few samp functions of AH(t). (b) Compute the mean function of PAH(t). (c) Compute the autocorrelation function RAH(t, 1). (d) Provide an integral expression for the characteristic function of AH (t). (e) Compute the 4th moment of AH (t). + 5 (f) Show that AH(t) is not a Gaussian random process by comparing the result of question 3d to the characteristic function of a Gaussian random variable having mean and variance identical to those of AH(t). (g) Show that AH(t) is not Gaussian by comparing the result of question 3e to the 4th moment of a Gaussian random variable having mean and variance identical to those of AH (t). 4. Let X(t) be a random process defined as: X(t) (A cos vt + B sin vt) + where are deterministic parameters, A and B are uncorrelated random variables for all 1 k (d) The helicopter explodes after smax hits. Define the lifetime of the helicopter as: m=1 Compute the expected value and variance of the lifetime. Hint: use the characteristic function. 9. Let A be a random variable with the following distribution: }={} for k= 1,2,3 otherwise Pr{A = k} = Define a random sequence {X} as: X = A, for all i > 1: . if if if Tlife Tm X = 1 X = 2 X = 3 Smax Y x - {2 Show that {Y} is not Markov. (a) Is {X} Markov? (b) Define the random sequence {Y} according to: Xi+1 = 2 Xi+1 3 Xi+1 1 1 if X = 1 2 otherwise