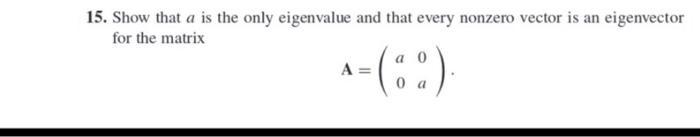
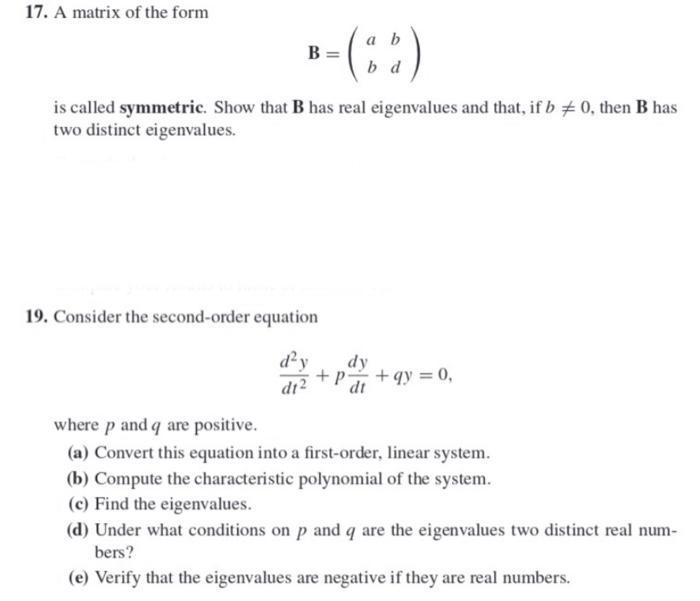
15. Show that a is the only eigenvalue and that every nonzero vector is an eigenvector for the matrix a A-(8%). = 0 a 17. A matrix of the form a b B-(8%) = b d is called symmetric. Show that B has real eigenvalues and that, if b 0, then B has two distinct eigenvalues. 19. Consider the second-order equation dy d2+p dy +qy = 0, where p and q are positive. (a) Convert this equation into a first-order, linear system. (b) Compute the characteristic polynomial of the system. (c) Find the eigenvalues. (d) Under what conditions on p and q are the eigenvalues two distinct real num- bers? (e) Verify that the eigenvalues are negative if they are real numbers.