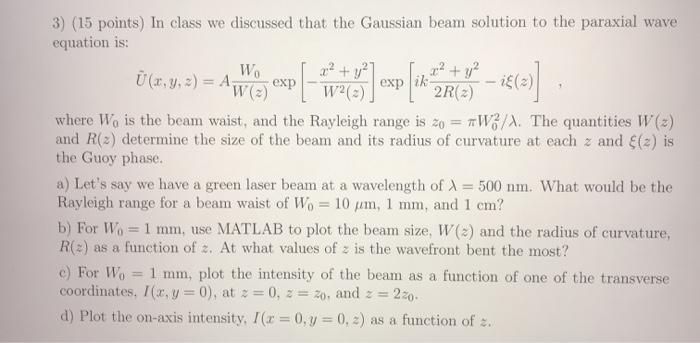
3) (15 points) In class we discussed that the Gaussian beam solution to the paraxial wave equation is: x + y] W(z) where Wo is the beam waist, and the Rayleigh range is zo W2/A. The quantities W(z) and R(2) determine the size of the beam and its radius of curvature at each z and (2) is the Guoy phase. Wo AW (2) exp P[-W/ (r, y, z) = A explik - ig(=)] x +y 2R(2) a) Let's say we have a green laser beam at a wavelength of A = 500 nm. What would be the Rayleigh range for a beam waist of Wo= 10 m, 1 mm, and 1 cm? b) For Wo= 1 mm, use MATLAB to plot the beam size, W(2) and the radius of curvature, R(2) as a function of 2. At what values of z is the wavefront bent the most? c) For Wo= 1 mm, plot the intensity of the beam as a function of one of the transverse coordinates, I(x, y=0), at z = 0, 2 = 20, and z = 2%0. d) Plot the on-axis intensity, I(r=0, y = 0, 2) as a function of 2.