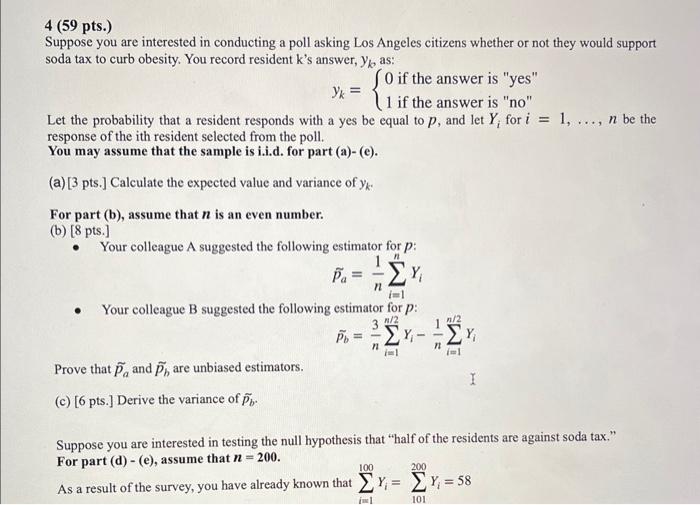
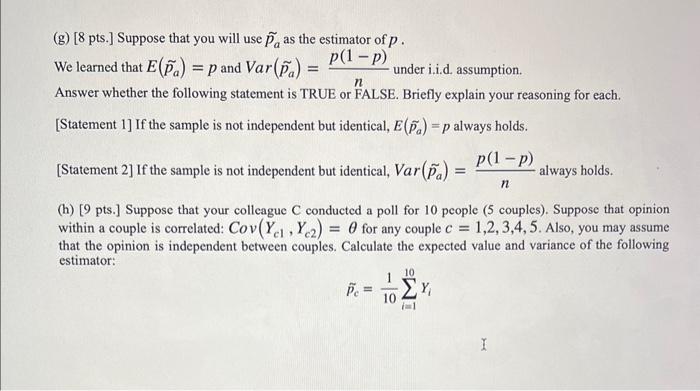
4 (59 pts.) Suppose you are interested in conducting a poll asking Los Angeles citizens whether or not they would support soda tax to curb obesity. You record resident k 's answer, yk as: yk={0iftheansweris"yes"1iftheansweris"no" Let the probability that a resident responds with a yes be equal to p, and let Yi for i=1,,n be the response of the ith resident selected from the poll. You may assume that the sample is i.i.d. for part (a)- (e). (a) [3 pts.] Calculate the expected value and variance of yk. For part (b), assume that n is an even number. (b) [8 pts.] - Your colleague A suggested the following estimator for p : p~a=n1i=1nYi - Your colleague B suggested the following estimator for p : pb~=n3i=1n/2Yin1i=1n/2Yi Prove that p~a and p~b are unbiased estimators. (c) [6pts. D Derive the variance of pb~. Suppose you are interested in testing the null hypothesis that "half of the residents are against soda tax." For part (d) - (e), assume that n=200. As a result of the survey, you have already known that i=1100Yi=101200Yi=58 Suppose you are interested in testing the null hypothesis that "half of the residents are against soda tax." For part (d) - (e), assume that n=200. As a result of the survey, you have already known that i=1100Yi=101200Yi=58 (d) (i) [7 pts.] Can you reject (with 5% size) the null hypothesis if you use pa~ as an estimator for p ? Clearly state the theorems you referred to, your steps, and your reasoning. Writing only the equations and your answer will not be given credits. (ii) [3 pts.] Derive 95% confidence interval associated with the estimator pa~. (e) (i) [7 pts.] Can you reject (with 5% size) the null hypothesis if you use p~h as an estimator for p ? Clearly state the theorems you referred to, your steps, and your reasoning. Writing only the equations and your answer will not be given credits. (ii) [3 pts.] Derive 95% confidence interval associated with the estimator pb~ (f) [5 pts.] Your colleague C (who is notorious for his laziness) suggested that "Why do not we ask the couples? We can finish the survey in half the time!!" Briefly explain why the independence of the sampling may be violated if we follow this suggestion. (g) [8 pts.] Suppose that you will use pa~ as the estimator of p. We learned that E(pa~)=p and Var(pa~)=np(1p) under i.i.d. assumption. Answer whether the following statement is TRUE or FALSE. Briefly explain your reasoning for each. [Statement 1] If the sample is not independent but identical, E(pa~)=p always holds. [Statement 2] If the sample is not independent but identical, Var(p~a)=np(1p) always holds. (h) [9 pts.] Suppose that your colleague C conducted a poll for 10 people ( 5 couples). Suppose that opinion within a couple is correlated: Cov(Yc1,Yc2)= for any couple c=1,2,3,4,5. Also, you may assume that the opinion is independent between couples. Calculate the expected value and variance of the following estimator: p~c=101i=110Yi 4 (59 pts.) Suppose you are interested in conducting a poll asking Los Angeles citizens whether or not they would support soda tax to curb obesity. You record resident k 's answer, yk as: yk={0iftheansweris"yes"1iftheansweris"no" Let the probability that a resident responds with a yes be equal to p, and let Yi for i=1,,n be the response of the ith resident selected from the poll. You may assume that the sample is i.i.d. for part (a)- (e). (a) [3 pts.] Calculate the expected value and variance of yk. For part (b), assume that n is an even number. (b) [8 pts.] - Your colleague A suggested the following estimator for p : p~a=n1i=1nYi - Your colleague B suggested the following estimator for p : pb~=n3i=1n/2Yin1i=1n/2Yi Prove that p~a and p~b are unbiased estimators. (c) [6pts. D Derive the variance of pb~. Suppose you are interested in testing the null hypothesis that "half of the residents are against soda tax." For part (d) - (e), assume that n=200. As a result of the survey, you have already known that i=1100Yi=101200Yi=58 Suppose you are interested in testing the null hypothesis that "half of the residents are against soda tax." For part (d) - (e), assume that n=200. As a result of the survey, you have already known that i=1100Yi=101200Yi=58 (d) (i) [7 pts.] Can you reject (with 5% size) the null hypothesis if you use pa~ as an estimator for p ? Clearly state the theorems you referred to, your steps, and your reasoning. Writing only the equations and your answer will not be given credits. (ii) [3 pts.] Derive 95% confidence interval associated with the estimator pa~. (e) (i) [7 pts.] Can you reject (with 5% size) the null hypothesis if you use p~h as an estimator for p ? Clearly state the theorems you referred to, your steps, and your reasoning. Writing only the equations and your answer will not be given credits. (ii) [3 pts.] Derive 95% confidence interval associated with the estimator pb~ (f) [5 pts.] Your colleague C (who is notorious for his laziness) suggested that "Why do not we ask the couples? We can finish the survey in half the time!!" Briefly explain why the independence of the sampling may be violated if we follow this suggestion. (g) [8 pts.] Suppose that you will use pa~ as the estimator of p. We learned that E(pa~)=p and Var(pa~)=np(1p) under i.i.d. assumption. Answer whether the following statement is TRUE or FALSE. Briefly explain your reasoning for each. [Statement 1] If the sample is not independent but identical, E(pa~)=p always holds. [Statement 2] If the sample is not independent but identical, Var(p~a)=np(1p) always holds. (h) [9 pts.] Suppose that your colleague C conducted a poll for 10 people ( 5 couples). Suppose that opinion within a couple is correlated: Cov(Yc1,Yc2)= for any couple c=1,2,3,4,5. Also, you may assume that the opinion is independent between couples. Calculate the expected value and variance of the following estimator: p~c=101i=110Yi