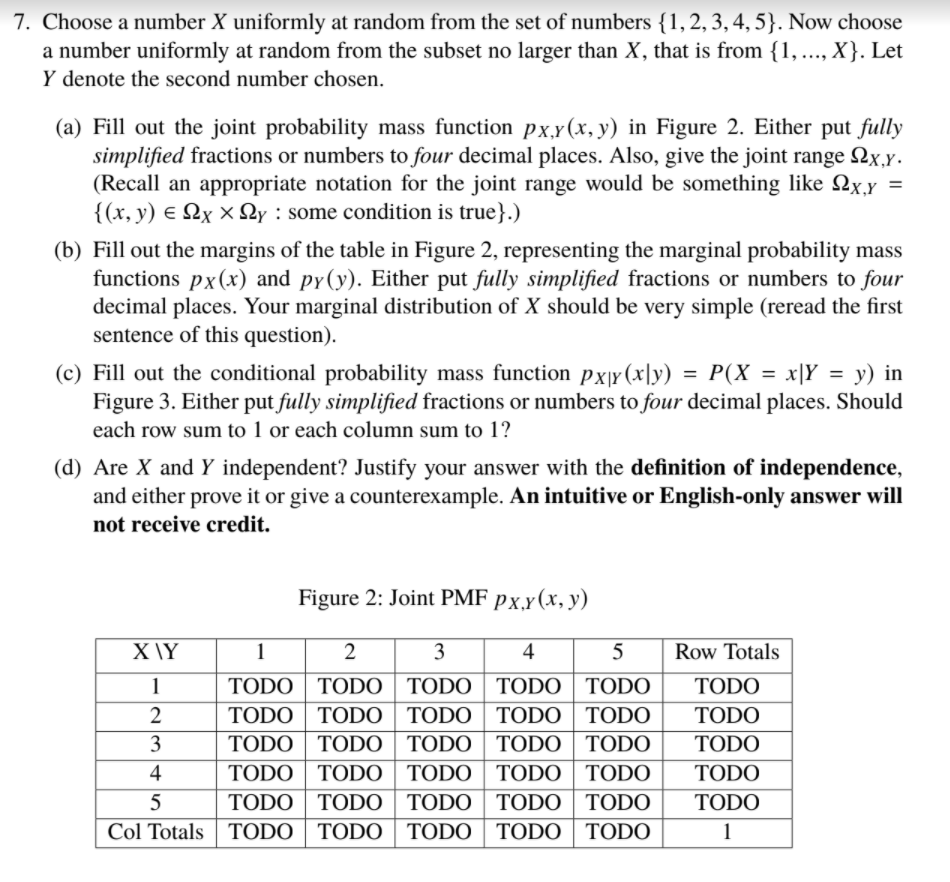
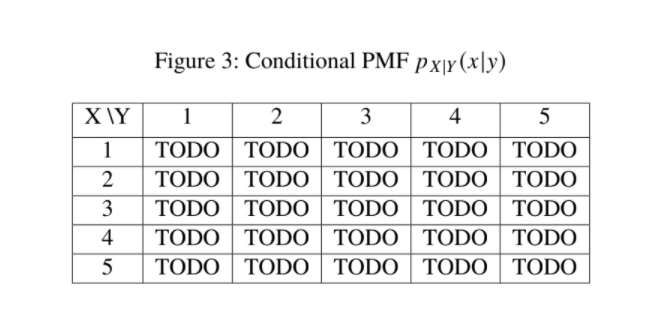
7. Choose a number X uniformly at random from the set of numbers {1,2,3,4,5). Now choose a number uniformly at random from the subset no larger than X, that is from {1,..., X}. Let Y denote the second number chosen. (a) Fill out the joint probability mass function px,y(x,y) in Figure 2. Either put fully simplified fractions or numbers to four decimal places. Also, give the joint range 12x,y. (Recall an appropriate notation for the joint range would be something like 2x,Y {(x, y) 12x x S2y : some condition is true}.) (b) Fill out the margins of the table in Figure 2, representing the marginal probability mass functions px(x) and py(y). Either put fully simplified fractions or numbers to four decimal places. Your marginal distribution of X should be very simple (reread the first sentence of this question). (c) Fill out the conditional probability mass function Pxy (x[y) = P(X = x\Y = y) in Figure 3. Either put fully simplified fractions or numbers to four decimal places. Should each row sum to 1 or each column sum to 1? (d) Are X and Y independent? Justify your answer with the definition of independence, and either prove it or give a counterexample. An intuitive or English-only answer will not receive credit. Figure 2: Joint PMF Px,y(x, y) 1 Row Totals 1 X Y 2 3 4 5 TODO TODO TODO TODO TODO 2 TODO TODO TODO TODO TODO 3 TODO TODO TODO TODO TODO 4 TODO TODO TODO TODO TODO 5 TODO TODO TODO TODO TODO Col Totals TODO TODO TODO TODO TODO TODO TODO TODO TODO TODO 1 Figure 3: Conditional PMF P x y(x|y) XY 1 1 2 3 4 2 3 4 5 TODO TODO TODO TODO TODO TODO TODO TODO TODO TODO TODO TODO TODO TODO TODO TODO TODO TODO TODO TODO TODO TODO TODO TODO TODO 5 7. Choose a number X uniformly at random from the set of numbers {1,2,3,4,5). Now choose a number uniformly at random from the subset no larger than X, that is from {1,..., X}. Let Y denote the second number chosen. (a) Fill out the joint probability mass function px,y(x,y) in Figure 2. Either put fully simplified fractions or numbers to four decimal places. Also, give the joint range 12x,y. (Recall an appropriate notation for the joint range would be something like 2x,Y {(x, y) 12x x S2y : some condition is true}.) (b) Fill out the margins of the table in Figure 2, representing the marginal probability mass functions px(x) and py(y). Either put fully simplified fractions or numbers to four decimal places. Your marginal distribution of X should be very simple (reread the first sentence of this question). (c) Fill out the conditional probability mass function Pxy (x[y) = P(X = x\Y = y) in Figure 3. Either put fully simplified fractions or numbers to four decimal places. Should each row sum to 1 or each column sum to 1? (d) Are X and Y independent? Justify your answer with the definition of independence, and either prove it or give a counterexample. An intuitive or English-only answer will not receive credit. Figure 2: Joint PMF Px,y(x, y) 1 Row Totals 1 X Y 2 3 4 5 TODO TODO TODO TODO TODO 2 TODO TODO TODO TODO TODO 3 TODO TODO TODO TODO TODO 4 TODO TODO TODO TODO TODO 5 TODO TODO TODO TODO TODO Col Totals TODO TODO TODO TODO TODO TODO TODO TODO TODO TODO 1 Figure 3: Conditional PMF P x y(x|y) XY 1 1 2 3 4 2 3 4 5 TODO TODO TODO TODO TODO TODO TODO TODO TODO TODO TODO TODO TODO TODO TODO TODO TODO TODO TODO TODO TODO TODO TODO TODO TODO 5








