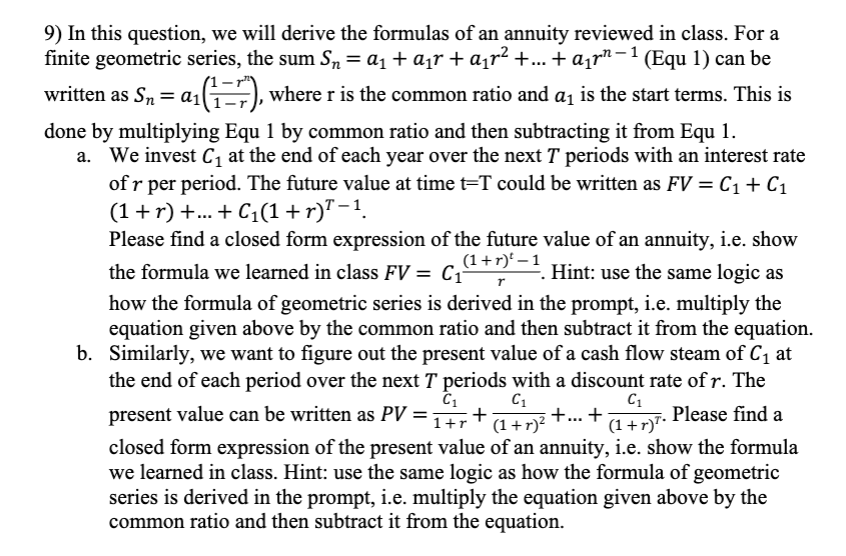
9) In this question, we will derive the formulas of an annuity reviewed in class. For a finite geometric series, the sum S = + ar + ar + ... + rn 1 (Equ 1) can be written as S = a(1777), where r is the common ratio and a is the start terms. This is Sn done by multiplying Equ 1 by common ratio and then subtracting it from Equ 1. a. We invest C at the end of each year over the next T periods with an interest rate of r per period. The future value at time t=T could be written as FV = C + C1 (1 + r) + ... + C(1+r) 1. Please find a closed form expression of the future value of an annuity, i.e. show the formula we learned in class FV = C(1+r)'. Hint: use the same logic as how the formula of geometric series is derived in the prompt, i.e. multiply the equation given above by the common ratio and then subtract it from the equation. b. Similarly, we want to figure out the present value of a cash flow steam of C at the end of each period over the next T periods with a discount rate of r. The C1 C1 present value can be written as PV = =1+r+ (1+r) +...+ C1 (1+r). Please find a closed form expression of the present value of an annuity, i.e. show the formula we learned in class. Hint: use the same logic as how the formula of geometric series is derived in the prompt, i.e. multiply the equation given above by the common ratio and then subtract it from the equation.