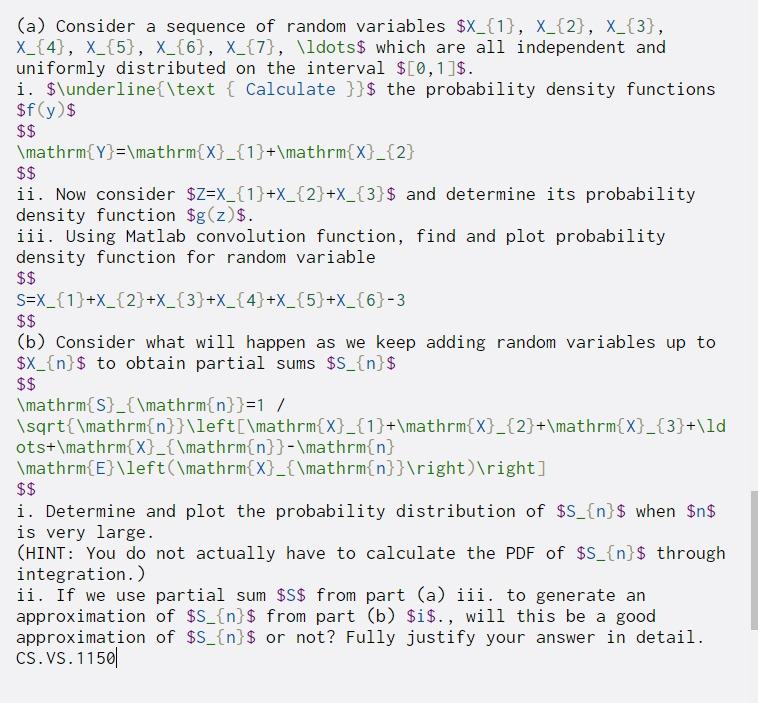
(a) Consider a sequence of random variables $X_{1}, X_{2}, X_{3}, X_{4}, X_{5}, X_{6}, X_{7}, \ldots which are all independent and uniformly distributed on the interval $[0,1]$. i. $\underline{\text { Calculate }}$ the probability density functions $f(y) $ $$ \mathrm{Y}=\mathrm{X}_{1}+\mathrm{X}_{2} $$ ii. Now consider $2=X_{1}+x_{2}+x_{3}$ and determine its probability density function $g(z)$. iii. Using Matlab convolution function, find and plot probability density function for random variable $$ SEX_{1}+x_{2}+x_{3}+x_{4}+x_{5}+x_{6}-3 $$ (b) Consider what will happen as we keep adding random variables up to $X_{n}$ to obtain partial sums $S_{n}$ $$ \mathrm{S}_{\mathrm{n}}=1 / \sqrt{\mathrm{n}}\left[\mathrm{X}_{1}+\mathrm{X} _{2}+\mathrm{X}_{3}+\ld ots+\mathrm{X}_{\mathrm{n}}-\mathrm{n} \mathrm{E}\left(\mathrm{X}_{\mathrm{n}} ight) ight] $$ i. Determine and plot the probability distribution of $S_{n} $ when $n$ is very large. (HINT: You do not actually have to calculate the PDF of $S_{n}$ through integration. ) ii. If we use partial sum $S$ from part (a) iii. to generate an approximation of $S_{n}$ from part (b) $i$., will this be a good approximation of $S_{n}$ or not? Fully justify your answer in detail. CS.VS. 1150 (a) Consider a sequence of random variables $X_{1}, X_{2}, X_{3}, X_{4}, X_{5}, X_{6}, X_{7}, \ldots which are all independent and uniformly distributed on the interval $[0,1]$. i. $\underline{\text { Calculate }}$ the probability density functions $f(y) $ $$ \mathrm{Y}=\mathrm{X}_{1}+\mathrm{X}_{2} $$ ii. Now consider $2=X_{1}+x_{2}+x_{3}$ and determine its probability density function $g(z)$. iii. Using Matlab convolution function, find and plot probability density function for random variable $$ SEX_{1}+x_{2}+x_{3}+x_{4}+x_{5}+x_{6}-3 $$ (b) Consider what will happen as we keep adding random variables up to $X_{n}$ to obtain partial sums $S_{n}$ $$ \mathrm{S}_{\mathrm{n}}=1 / \sqrt{\mathrm{n}}\left[\mathrm{X}_{1}+\mathrm{X} _{2}+\mathrm{X}_{3}+\ld ots+\mathrm{X}_{\mathrm{n}}-\mathrm{n} \mathrm{E}\left(\mathrm{X}_{\mathrm{n}} ight) ight] $$ i. Determine and plot the probability distribution of $S_{n} $ when $n$ is very large. (HINT: You do not actually have to calculate the PDF of $S_{n}$ through integration. ) ii. If we use partial sum $S$ from part (a) iii. to generate an approximation of $S_{n}$ from part (b) $i$., will this be a good approximation of $S_{n}$ or not? Fully justify your answer in detail. CS.VS. 1150