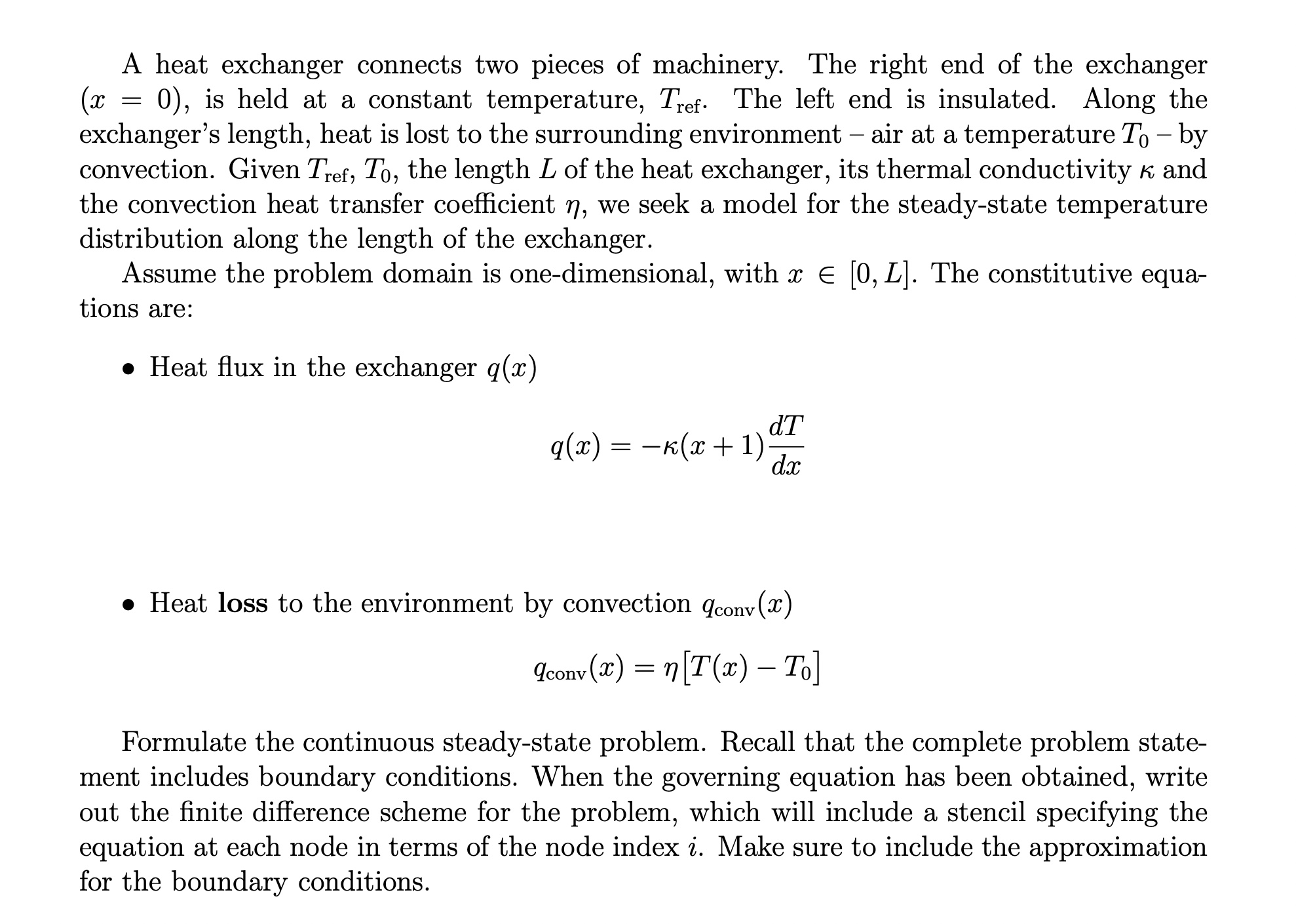
A heat exchanger connects two pieces of machinery. The right end of the exchanger (x = 0), is held at a constant temperature, Tref. The left end is insulated. Along the exchanger's length, heat is lost to the surrounding environment - air at a temperature To - by convection. Given Tref, To, the length L of the heat exchanger, its thermal conductivity and the convection heat transfer coefficient n, we seek a model for the steady-state temperature distribution along the length of the exchanger. Assume the problem domain is one-dimensional, with [0,L]. The constitutive equa- tions are: Heat flux in the exchanger q(x) dT q(x) = k(x + 1) k(x+1) dx Heat loss to the environment by convection conv(x) Iconv(x) = n[T(x) To] - Formulate the continuous steady-state problem. Recall that the complete problem state- ment includes boundary conditions. When the governing equation has been obtained, write out the finite difference scheme for the problem, which will include a stencil specifying the equation at each node in terms of the node index i. Make sure to include the approximation for the boundary conditions.