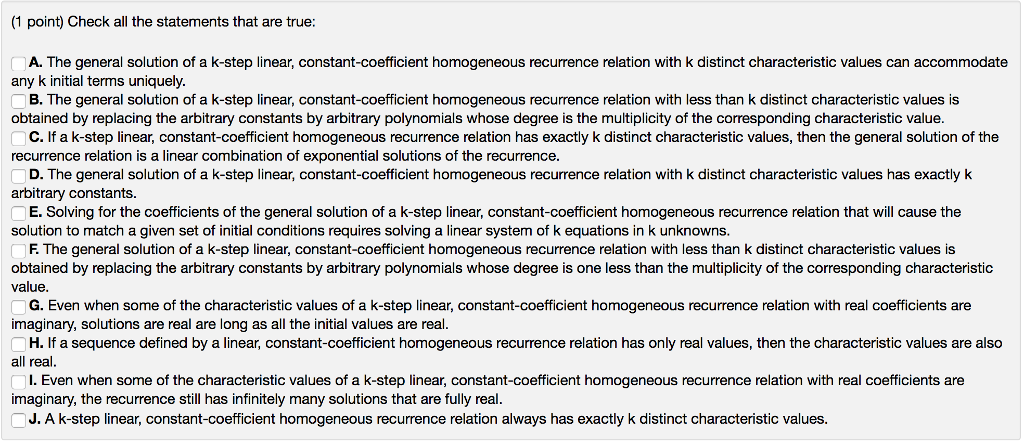
Check all the statements that are true: The general solution of a k-step linear, constant-coefficient homogeneous recurrence relation with k distinct characteristic values can accommodate any k initial terms uniquely. The general solution of a k-step linear, constant-coefficient homogeneous recurrence relation with less than k distinct characteristic values is obtained by replacing the arbitrary constants by arbitrary polynomials whose degree is the multiplicity of the corresponding characteristic value. If a k-step linear, constant-coefficient homogeneous recurrence relation has exactly k distinct characteristic values, then the general solution of the recurrence relation is a linear combination of exponential solutions of the recurrence. The general solution of a k-step linear, constant-coefficient homogeneous recurrence relation with k distinct characteristic values has exactly k arbitrary constants. Solving for the coefficients of the general solution of a k-step linear, constant-coefficient homogeneous recurrence relation that will cause the solution to match a given set of initial conditions requires solving a linear system of k equations in k unknowns. The general solution of a k-step linear, constant-coefficient homogeneous recurrence relation with less than k distinct characteristic values is obtained by replacing the arbitrary constants by arbitrary polynomials whose degree is one less than the multiplicity of the corresponding characteristic value. Even when some of the characteristic values of a k-step linear, constant-coefficient homogeneous recurrence relation with real coefficients are imaginary, solutions are real are long as all the initial values are real. If a sequence defined by a linear, constant-coefficient homogeneous recurrence relation has only real values, then the characteristic values are also all real. Even when some of the characteristic values of a k-step linear, constant-coefficient homogeneous recurrence relation with real coefficients are imaginary, the recurrence still has infinitely many solutions that are fully real. A k-step linear, constant-coefficient homogeneous recurrence relation always has exactly k distinct characteristic values