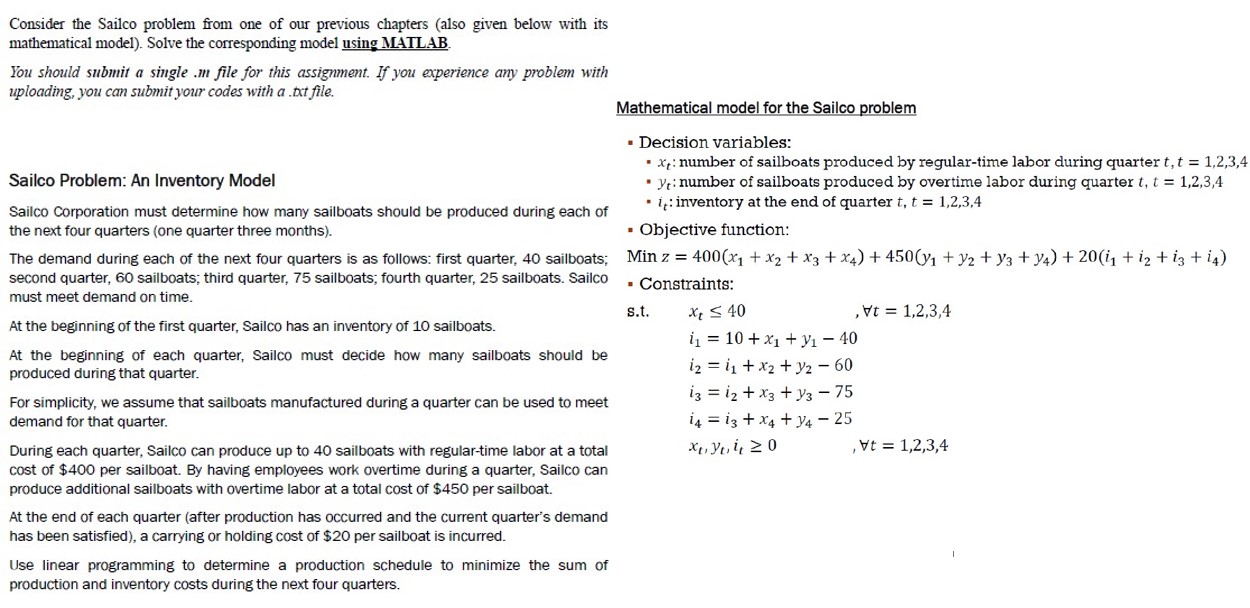Consider the Sailco problem from one of our previous chapters (also given below with its mathematical model). Solve the corresponding model using MATLAB. You should submit a single .m file for this assignment. If you experience any problem with uploading, you can submit your codes with a txt file. Mathematical model for the Sailco problem - Decision variables: - xt: number of sailboats produced by regular-time labor during quarter t,t=1,2,3,4 - yt : number of sailboats produced by overtime labor during quarter t,t=1,2,3,4 - it : inventory at the end of quarter t,t=1,2,3,4 Sailco Corporation must determine how many sailboats should be produced during each of the next four quarters (one quarter three months). The demand during each of the next four quarters is as follows: first quarter, 40 sailboats; second quarter, 60 sailboats; third quarter, 75 sailboats; fourth quarter, 25 sailboats. Sailco must meet demand on time. At the beginning of the first quarter, Sailco has an inventory of 10 sailboats. At the beginning of each quarter, Sailco must decide how many sailboats should be produced during that quarter. For simplicity, we assume that sailboats manufactured during a quarter can be used to meet demand for that quarter. During each quarter, Sailco can produce up to 40 sailboats with regular-time labor at a total cost of $400 per sailboat. By having employees work overtime during a quarter, Sailco can produce additional sailboats with overtime labor at a total cost of $450 per sailboat. At the end of each quarter (after production has occurred and the current quarter's demand has been satisfied), a carrying or holding cost of $20 per sailboat is incurred. Use linear programming to determine a production schedule to minimize the sum of - Objective function: Minz=400(x1+x2+x3+x4)+450(y1+y2+y3+y4)+20(i1+i2+i3+i4) - Constraints: s.t.i3=i2+x3+y375i4=i3+x4+y425xt,yt,it0,t=1,2,3,4xt40,t=1,2,3,4i1=10+x1+y140i2=i1+x2+y260 production and inventory costs during the next four quarters