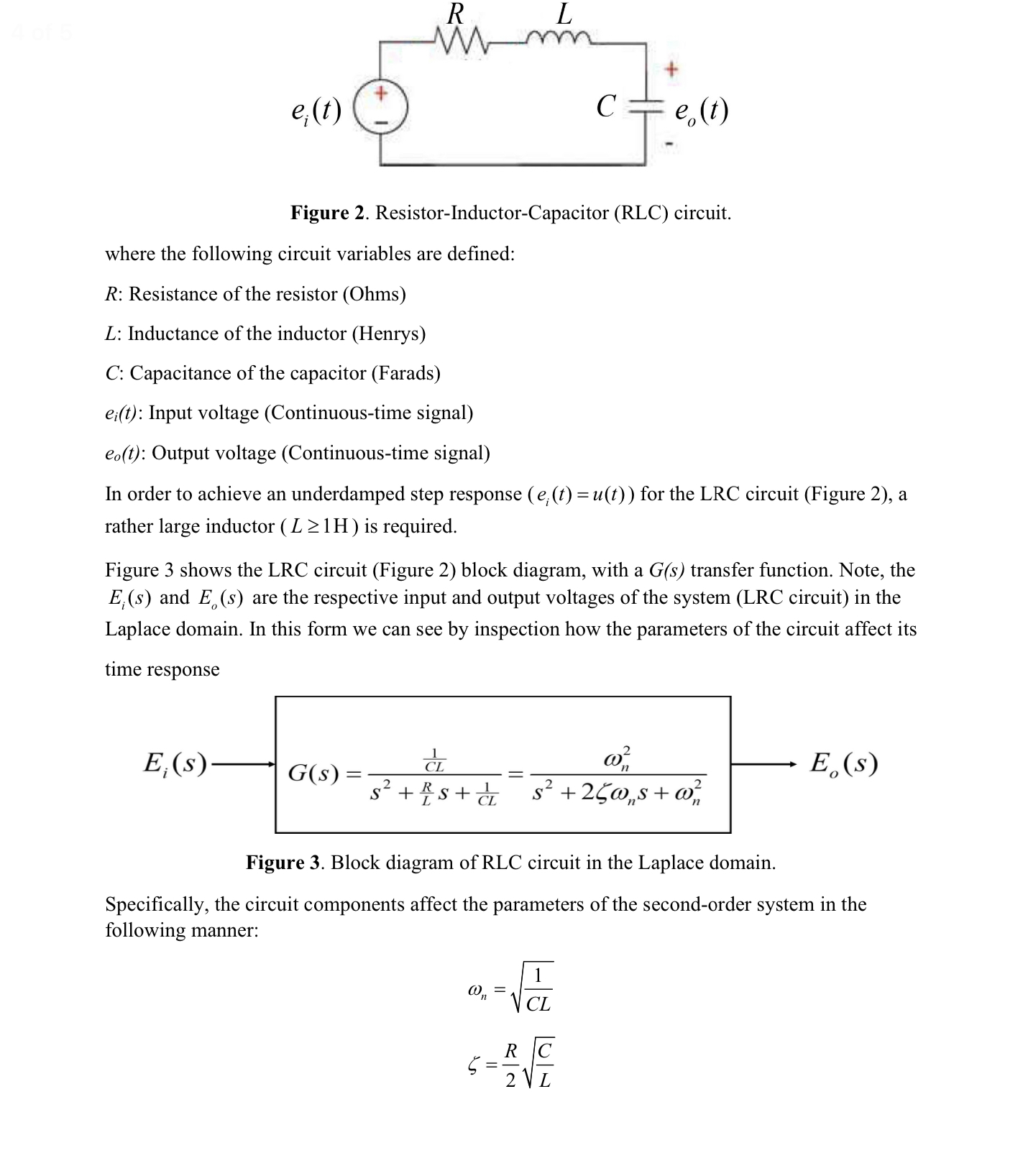
e(t) R L C e(t) Figure 2. Resistor-Inductor-Capacitor (RLC) circuit. where the following circuit variables are defined: R: Resistance of the resistor (Ohms) L: Inductance of the inductor (Henrys) C: Capacitance of the capacitor (Farads) ei(t): Input voltage (Continuous-time signal) eo(t): Output voltage (Continuous-time signal) In order to achieve an underdamped step response (e,(t) = u(t)) for the LRC circuit (Figure 2), a rather large inductor (L1H) is required. Figure 3 shows the LRC circuit (Figure 2) block diagram, with a G(s) transfer function. Note, the E(s) and E(s) are the respective input and output voltages of the system (LRC circuit) in the Laplace domain. In this form we can see by inspection how the parameters of the circuit affect its time response E,(s) ? G(s) = CL n 2 s + s +25ws+w E(s) Figure 3. Block diagram of RLC circuit in the Laplace domain. Specifically, the circuit components affect the parameters of the second-order system in the following manner: 1 @n CL RC S 2VL where the natural frequency @, and damping ratio 5 parameters relate to the characteristics of an underdamped second-order step response. Tasks: n 1. Determine the poles of the transfer function G(s) for the values of 5 and @, in Table 1. 2. Calculate by hand the overshoot (PO) and peak time (tp) step response of the (LRC) circuit for the values of 5 and @ in Table 1. 3. Using the MATLAB script below and the values of 5 and @, in Table 1, plot the step response of the RLC circuit. Graphically estimate the overshoot (PO) and peak-time using MATLAB tools (Hint: Data cursor). num=??; den =??; y= tf(num,den); %Transfer function of the system stepplot(y) %Step response 4. Using Simulink, build and simulate the step response of the RLC circuit block diagram (Figure 3). a. Start a new Simulink model window, drag the following blocks from the Simulink library window: Step (from Sources), Transfer Function (from Continuous), Scope (from Sinks), and Save File to Workspace (from Sinks). b. Arrange and connect the blocks. c. Enter blocks' parameters values. Use the values of 5 and w, in Table 1. d. Deliverable: Simulink model and plots of step responses. Table 1. Transfer function parameters for varying time constants (seconds) (rad/s) Poles (s=?) 'n Calculated Estimated (MATLAB) PO(%) tp (seconds) PO(%) tp (seconds) 0.1 5 0.05 10 0.1 100 5. Discuss your observations of the step response when varying the natural frequency and the damping ratio.