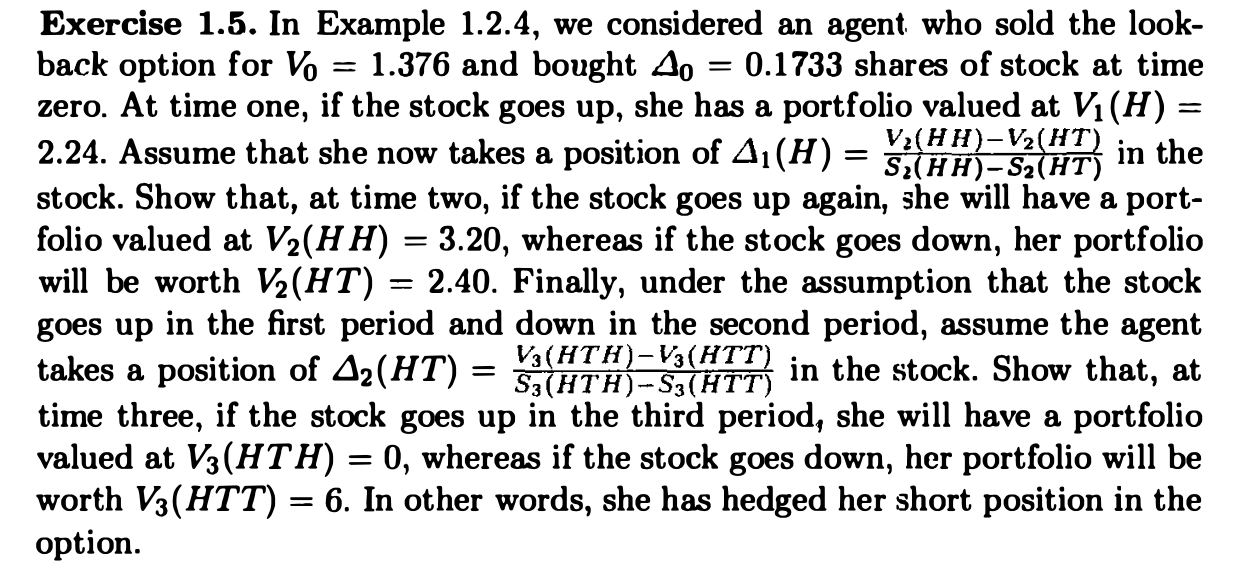
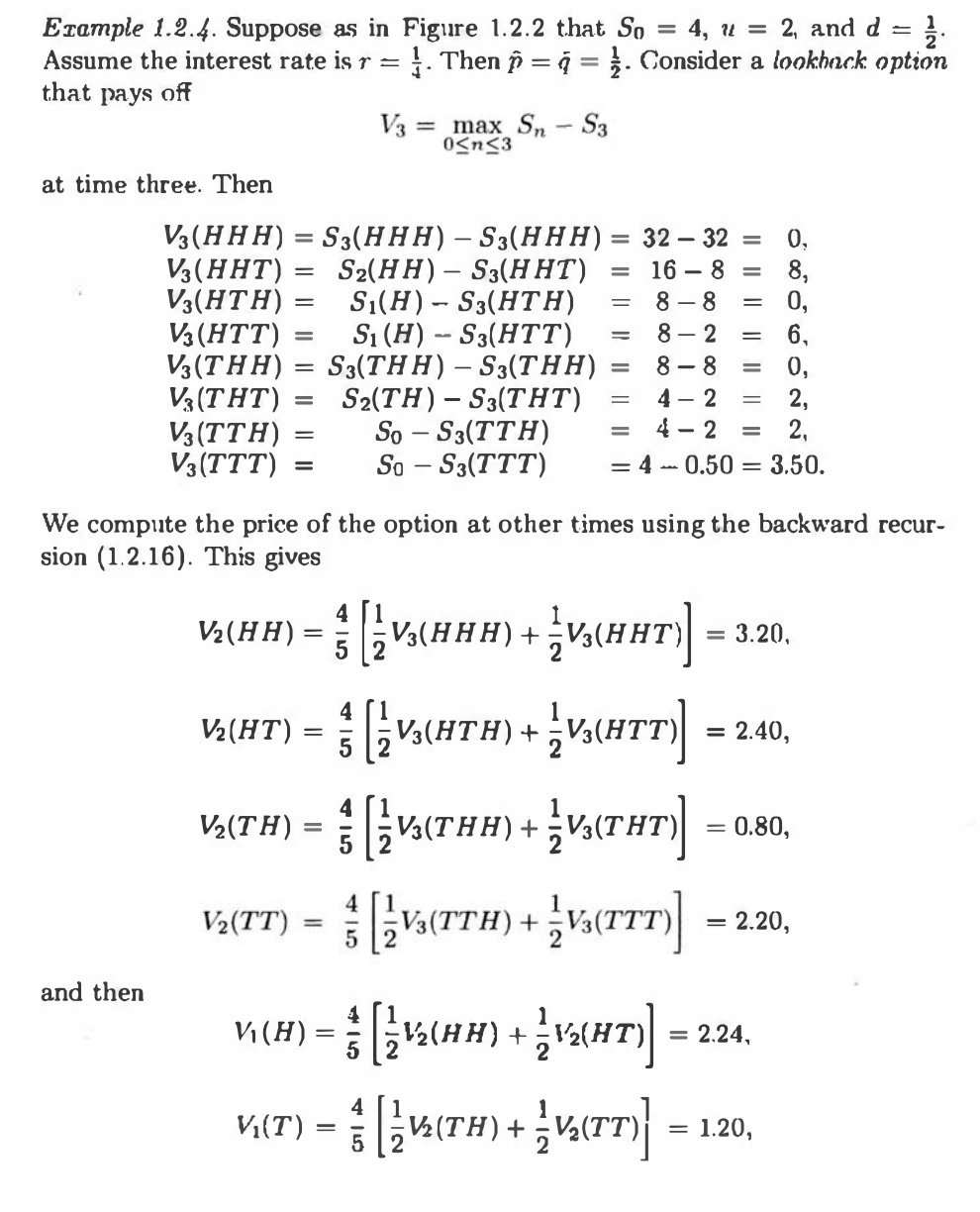
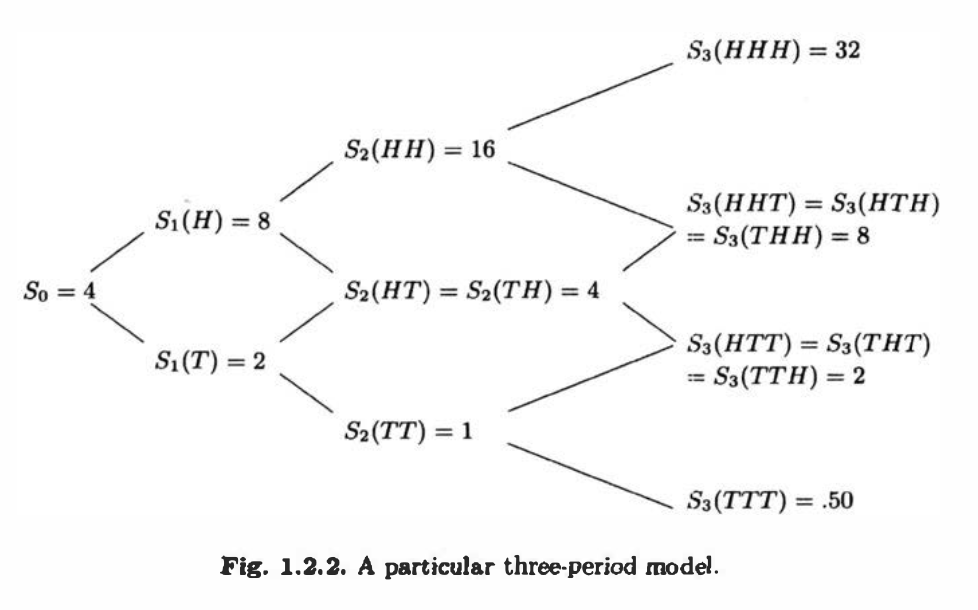
= = Exercise 1.5. In Example 1.2.4, we considered an agent who sold the look- back option for Vo 1.376 and bought 40 0.1733 shares of stock at time zero. At time one, if the stock goes up, she has a portfolio valued at V (H) = 2.24. Assume that she now takes a position of A(H) = V(HH)V2(HT) in the S(HH)-S2(HT) stock. Show that, at time two, if the stock goes up again, she will have a port- folio valued at V(HH) = 3.20, whereas if the stock goes down, her portfolio will be worth V(HT) = 2.40. Finally, under the assumption that the stock goes up in the first period and down in the second period, assume the agent V (HTH) V3(HTT) takes a position of 42 (HT) = 53(HTH) -S3(HTT) in the stock. Show that, at time three, if the stock goes up in the third period, she will have a portfolio valued at V3 (HTH) = 0, whereas if the stock goes down, her portfolio will be worth V3(HTT) = 6. In other words, she has hedged her short position in the option. = Example 1.2.4. Suppose as in Figure 1.2.2 that So = 4, u = 2, and d Assume the interest rate is r = 1. Then y = q = . Consider a lookback option that pays off at time three. Then V3 max Sn - S3 0n3 S3(HHH) - S3(HHH) = 32 - 32 = = 0. || || | 16-8 = 8-8 8, = 0, V3(HHH) V3(HHT) V3(HTH) S2(HH) S3(HHT) = = S(H) S3(HTH) V3 (HTT) = S(H) S3(HTT) = 8-2 = 6, V3(THH) = S3(THH) - S3(THH) = 8-8 = 0, V3 (THT) = S2(TH) S3(THT) 4-2 = 2, V3 (TTH) = So - S3(TTH) = 4-2 = 2 So-S3(TTT) - = 4 0.50 3.50. = V3 (TTT) = We compute the price of the option at other times using the backward recur- sion (1.2.16). This gives 4 V(HH): = (HHH) + (HHT)] = 3.20, 4 V(HT) = 5 V(HT) + (HTT)] = 2.40, V(TH) = [ (THH) + (THT)] = = 0.80, and then V(TT) = Va(TTH) + V(TTT)] [3 V (H) = [ { (HH) + 2(HT)] = = 2.20, = 2.24, V(T) = [V(TH) + V(TT) = 1.20, S3(HHH)=32 S(H)=8 So = 4 S(T) = 2 S2(HH) = 16 = S2(HT) S2(TH) = 4 S3(HHT) =S3(HTH) = S3(THH) = 8 S3(HTT) =S3(THT) ==== S3(TTH) = 2 S2 (TT) = 1 S3 (TTT): = .50 Fig. 1.2.2. A particular three-period model.