Financial Risk Modelling

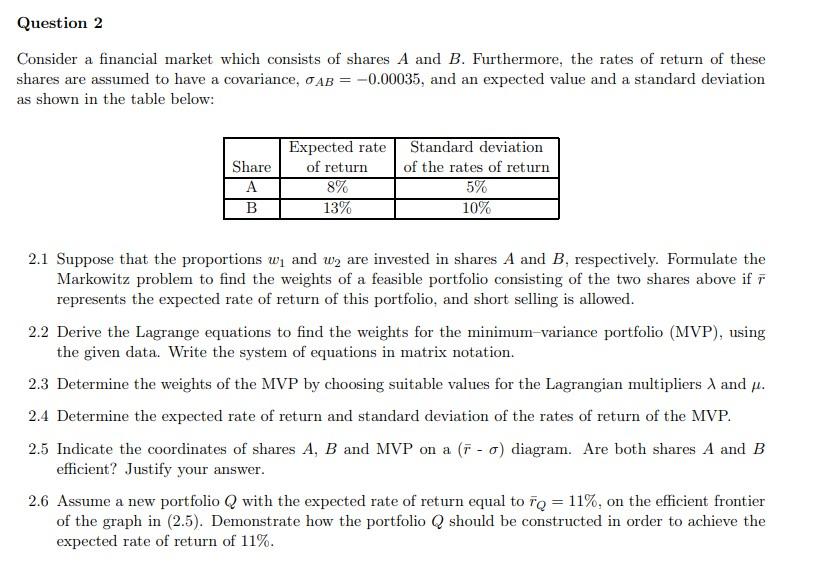
Question 2 Consider a financial market which consists of shares A and B. Furthermore, the rates of return of these shares are assumed to have a covariance, AB = -0.00035, and an expected value and a standard deviation as shown in the table below: Share A Expected rate of return 8% 13% Standard deviation of the rates of return 5% 10% B 2.1 Suppose that the proportions w, and w, are invested in shares A and B, respectively. Formulate the Markowitz problem to find the weights of a feasible portfolio consisting of the two shares above if represents the expected rate of return of this portfolio, and short selling is allowed. 2.2 Derive the Lagrange equations to find the weights for the minimum-variance portfolio (MVP), using the given data. Write the system of equations in matrix notation. 2.3 Determine the weights of the MVP by choosing suitable values for the Lagrangian multipliers , and H. 2.4 Determine the expected rate of return and standard deviation of the rates of return of the MVP. 2.5 Indicate the coordinates of shares A, B and MVP on a (i - o) diagram. Are both shares A and B efficient? Justify your answer. 2.6 Assume a new portfolio Q with the expected rate of return equal to fo = 11%, on the efficient frontier of the graph in (2.5). Demonstrate how the portfolio should be constructed in order to achieve the expected rate of return of 11%. Question 2 Consider a financial market which consists of shares A and B. Furthermore, the rates of return of these shares are assumed to have a covariance, AB = -0.00035, and an expected value and a standard deviation as shown in the table below: Share A Expected rate of return 8% 13% Standard deviation of the rates of return 5% 10% B 2.1 Suppose that the proportions w, and w, are invested in shares A and B, respectively. Formulate the Markowitz problem to find the weights of a feasible portfolio consisting of the two shares above if represents the expected rate of return of this portfolio, and short selling is allowed. 2.2 Derive the Lagrange equations to find the weights for the minimum-variance portfolio (MVP), using the given data. Write the system of equations in matrix notation. 2.3 Determine the weights of the MVP by choosing suitable values for the Lagrangian multipliers , and H. 2.4 Determine the expected rate of return and standard deviation of the rates of return of the MVP. 2.5 Indicate the coordinates of shares A, B and MVP on a (i - o) diagram. Are both shares A and B efficient? Justify your answer. 2.6 Assume a new portfolio Q with the expected rate of return equal to fo = 11%, on the efficient frontier of the graph in (2.5). Demonstrate how the portfolio should be constructed in order to achieve the expected rate of return of 11%