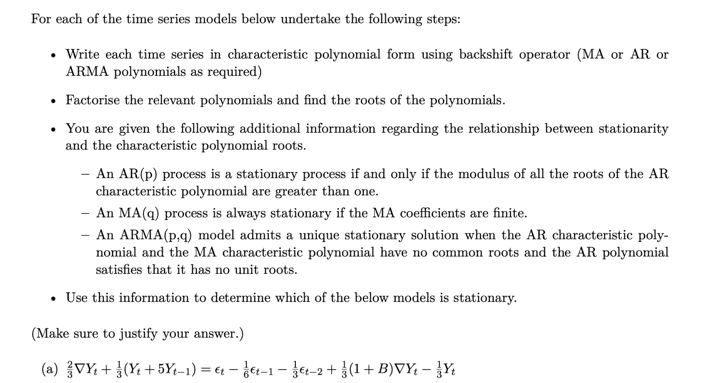
For each of the time series models below undertake the following steps: Write each time series in characteristic polynomial form using backshift operator (MA or AR or ARMA polynomials as required) Factorise the relevant polynomials and find the roots of the polynomials. You are given the following additional information regarding the relationship between stationarity and the characteristic polynomial roots. -An AR(p) process is a stationary process if and only if the modulus of all the roots of the AR characteristic polynomial are greater than one. - An MA(q) process is always stationary if the MA coefficients are finite. -An ARMA (p,q) model admits a unique stationary solution when the AR characteristic poly- nomial and the MA characteristic polynomial have no common roots and the AR polynomial satisfies that it has no unit roots. Use this information to determine which of the below models is stationary. (Make sure to justify your answer.) (a) VY+(X+5Y-1) = &t-et-1-et-2 + (1 + B)VY - Y