Answered step by step
Verified Expert Solution
Question
1 Approved Answer
Hi, I am confused about how to do the conversions for these three problems and how to find the bounds of each integral after making
Hi, I am confused about how to do the conversions for these three problems and how to find the bounds of each integral after making the conversions. Can someone please help walk me through those processes?
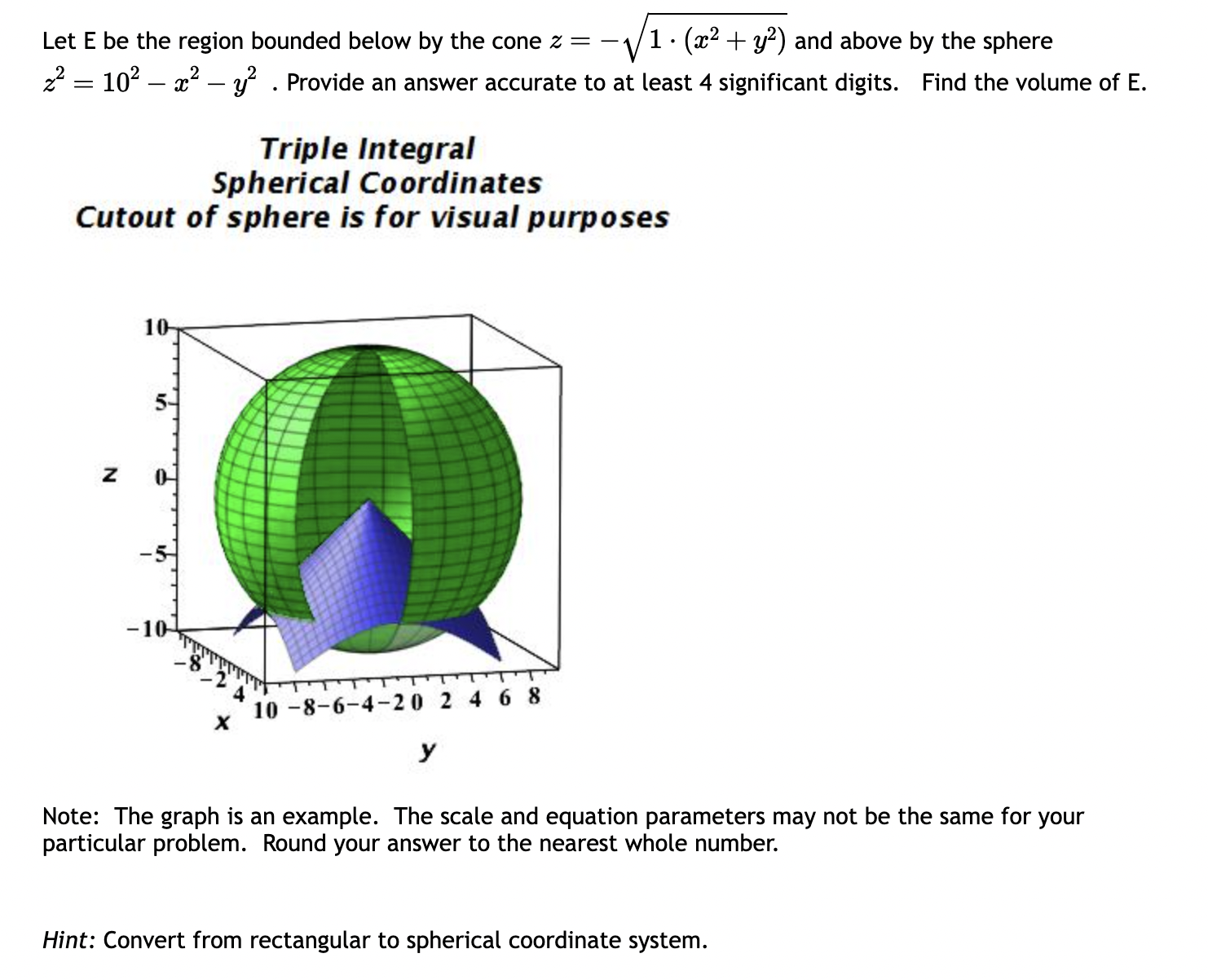
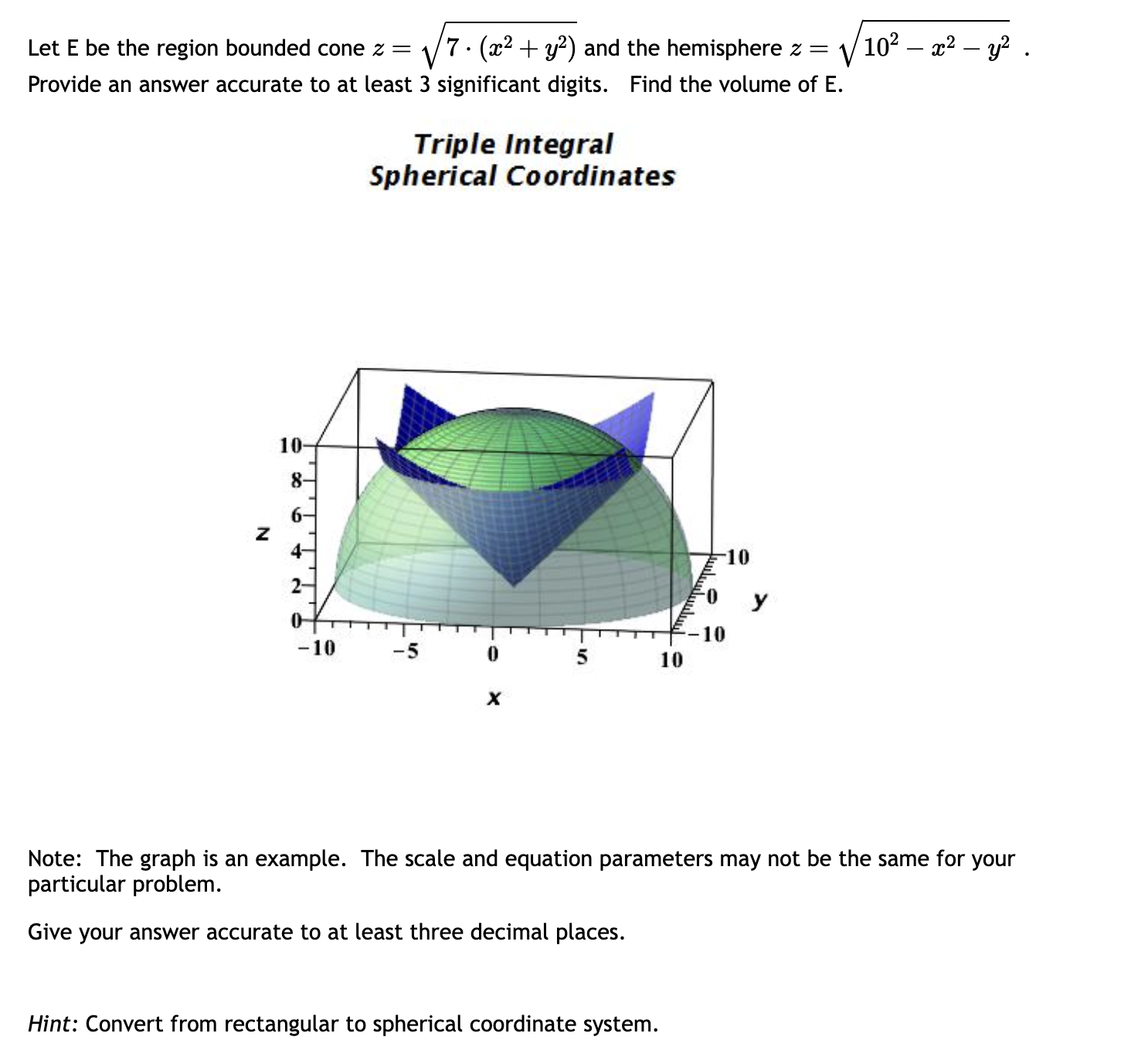
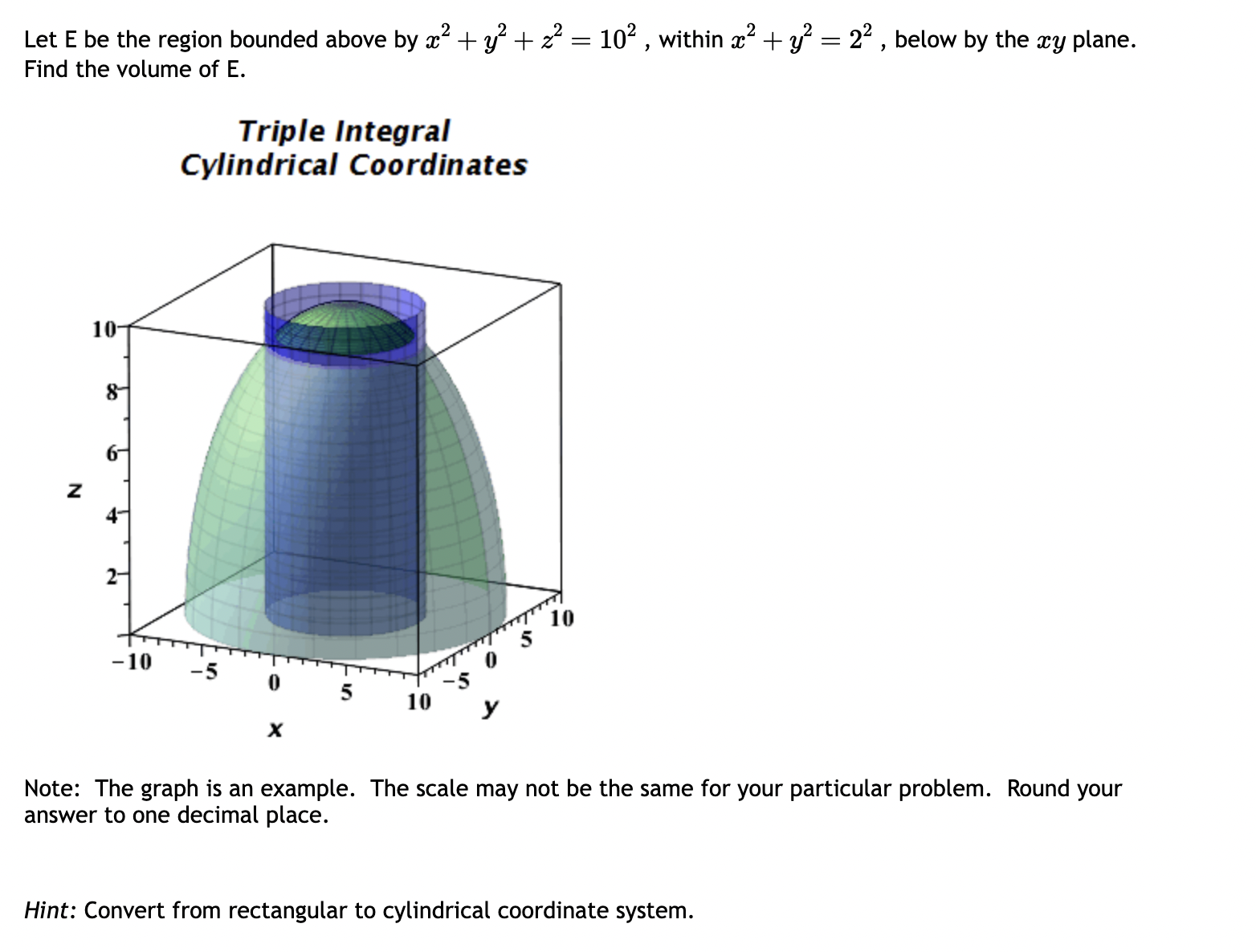
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


