Question
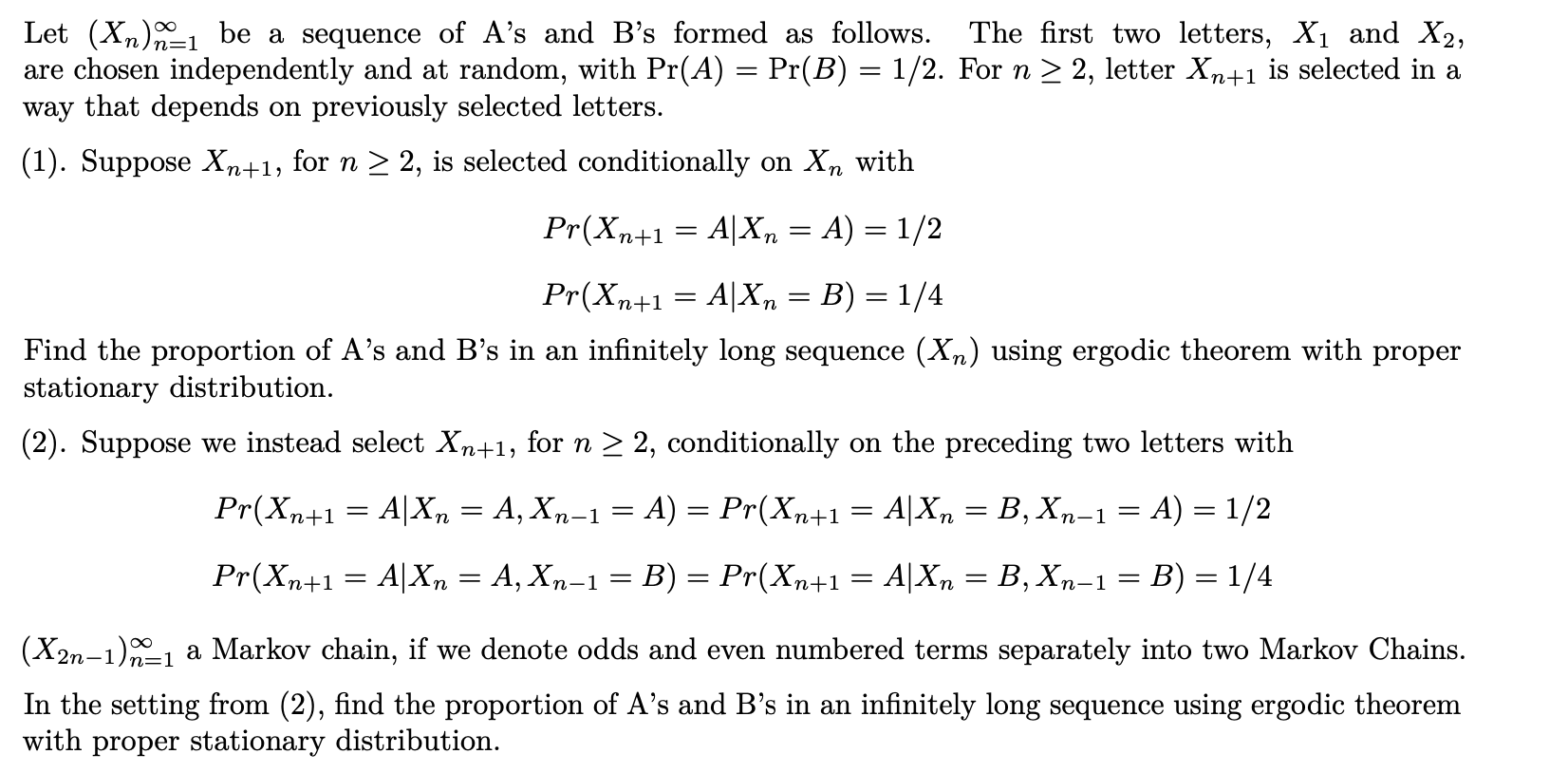
Let (X_n)^infty_{n=1} be a sequence of A's and B's formed as follows. The first two letters, X_1 and X_2, are chosen independently and at random,
Let (X_n)^\infty_{n=1} be a sequence of A's and B's formed as follows. The first two letters, X_1 and X_2, are chosen independently and at random, with Pr(A) = Pr(B) = 1/2. For n \geq 2 (i.e. n>=2), letter X_{n+1} is selected in a way that depends on previously selected letters.
(1). Suppose X_{n+1}, for n \geq 2 (i.e. n>=2) , is selected conditionally on X_n with
Pr(X_{n+1} =A|X_n =A)=1/2
Pr(X_{n+1} =A|X_n =B)=1/4
Find the proportion of A's and B's in an infinitely long sequence (X_n) using ergodic theorem with proper stationary distribution.
(2). Suppose we instead select X_{n+1}, for n \geq 2, conditionally on the preceding two letters with
Pr(X_{n+1} = A|X_n = A,X_{n-1} = A) = Pr(X_{n+1} = A|X_n = B,X_{n-1} = A) = 1/2
Pr(X_{n+1} = A|X_n = A,X_{n-1} = B) = Pr(X_{n+1} = A|X_n = B,X_{n-1} = B) = 1/4
(X_{2n-1})^\infty _{n=1} is a Markov chain if we denote odds and even numbered terms separately into two Markov Chains.
In the setting from (2), find the proportion of A's and B's in an infinitely long sequence using ergodic theorem with proper stationary distribution.
(See more details of the equations and sequences in the snapshot. Same content)
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


