(Machine Learning) Serious answer only please, better with explanation, thanks !

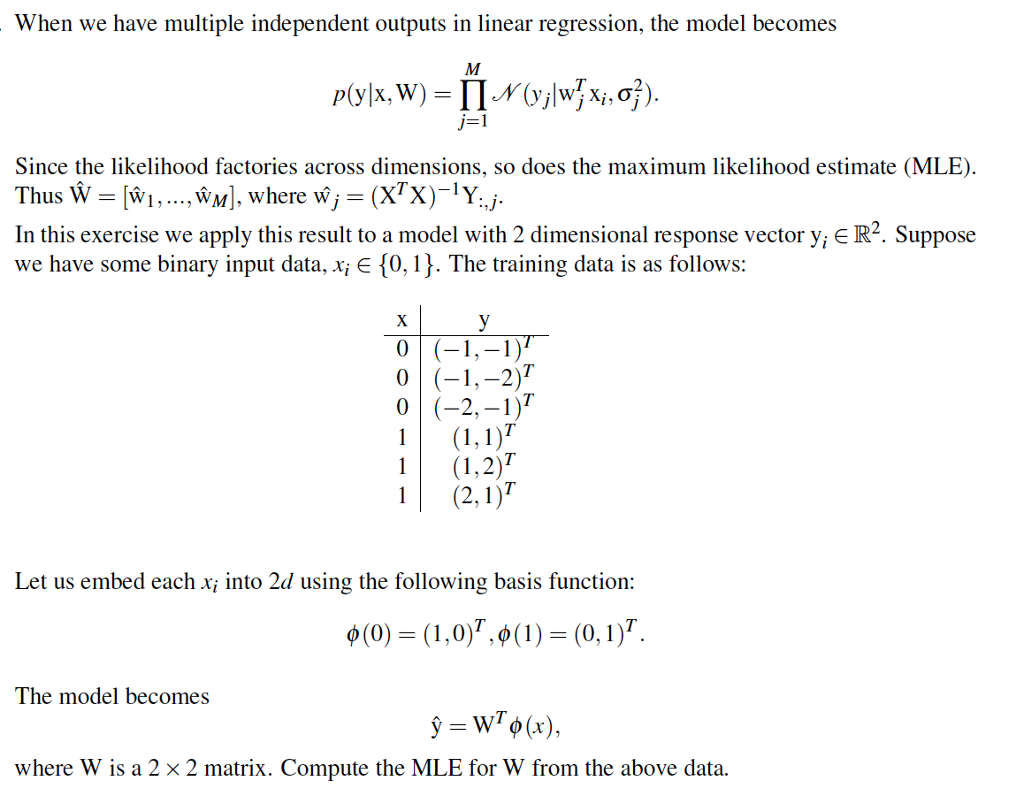
When we have multiple independent outputs in linear regression, the model becomes Since the likelihood factories across dimensions, so does the maximum likelihood estimate (MLE) Thus w = [wi , , wM), where w,-(XTX)-1 Y.J In this exercise we apply this result to a model with 2 dimensional response vector y, E R2. Suppose we have some binary input data, x; E 10, 1). The training data is as follows: Let us embed each xi into 2d using the following basis function: The model becomes where W is a 2x 2 matrix. Compute the MLE for W from the above data When we have multiple independent outputs in linear regression, the model becomes Since the likelihood factories across dimensions, so does the maximum likelihood estimate (MLE) Thus w = [wi , , wM), where w,-(XTX)-1 Y.J In this exercise we apply this result to a model with 2 dimensional response vector y, E R2. Suppose we have some binary input data, x; E 10, 1). The training data is as follows: Let us embed each xi into 2d using the following basis function: The model becomes where W is a 2x 2 matrix. Compute the MLE for W from the above data