Answered step by step
Verified Expert Solution
Question
1 Approved Answer
MATLAB CODE ANSWERES NEEDED FOR: (a) Solve the problem using fourth-order Runge-Kutta method. (b) Solve the problem using one of the MATLABs built-in functions for
MATLAB CODE ANSWERES NEEDED FOR:
(a) Solve the problem using fourth-order Runge-Kutta method.
(b) Solve the problem using one of the MATLABs built-in functions for solving ODE.
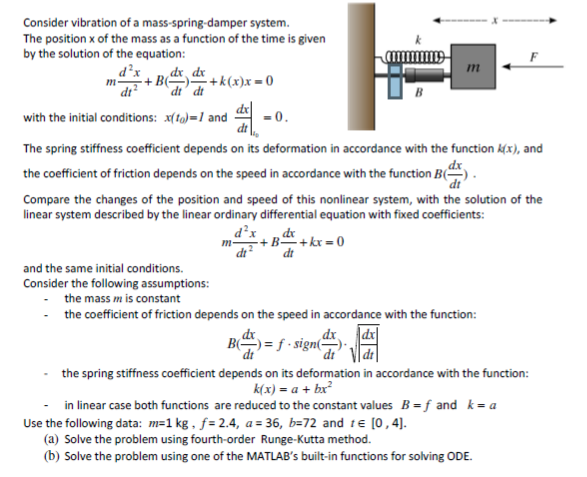
Consider vibration of a mass-spring-damper system. The position x of the mass as a function of the time is given by the solution of the equation: dt dt with the initial conditions: (to)-1 and The spring stiffness coefficient depends on its deformation in accordance with the function Afx), and the coefficient of friction depends on the speed in accordance with the function B Compare the changes of the position and speed of this nonlinear system, with the solution of the dt dt linear system described by the linear ordinary differential equation with fixed coefficients: dt dt and the same initial conditions. Consider the following assumptions: - the mass m is constant - the coefficient of friction depends on the speed in accordance with the function: dx dx dt dtVd the spring stiffness coefficient depends on its deformation in accordance with the function: - k(x) ba in linear case both functions are reduced to the constant values B-f and ka Use the following data: m-1kg, f- 2.4, a-36, b-72 and e [0,4] (a) Solve the problem using fourth-order Runge-Kutta method. (b) Solve the problem using one of the MATLAB's built-in functions for solving ODE. Consider vibration of a mass-spring-damper system. The position x of the mass as a function of the time is given by the solution of the equation: dt dt with the initial conditions: (to)-1 and The spring stiffness coefficient depends on its deformation in accordance with the function Afx), and the coefficient of friction depends on the speed in accordance with the function B Compare the changes of the position and speed of this nonlinear system, with the solution of the dt dt linear system described by the linear ordinary differential equation with fixed coefficients: dt dt and the same initial conditions. Consider the following assumptions: - the mass m is constant - the coefficient of friction depends on the speed in accordance with the function: dx dx dt dtVd the spring stiffness coefficient depends on its deformation in accordance with the function: - k(x) ba in linear case both functions are reduced to the constant values B-f and ka Use the following data: m-1kg, f- 2.4, a-36, b-72 and e [0,4] (a) Solve the problem using fourth-order Runge-Kutta method. (b) Solve the problem using one of the MATLAB's built-in functions for solving ODE
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


