please help
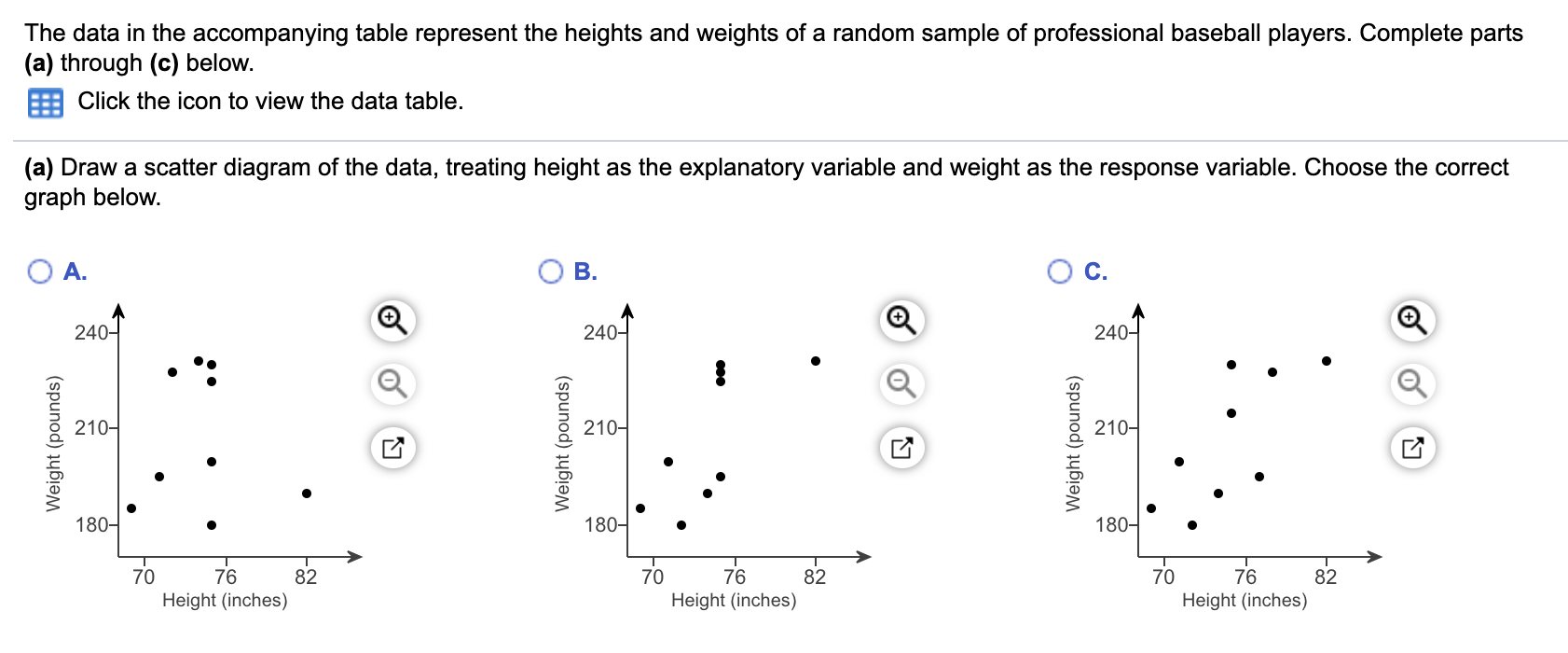
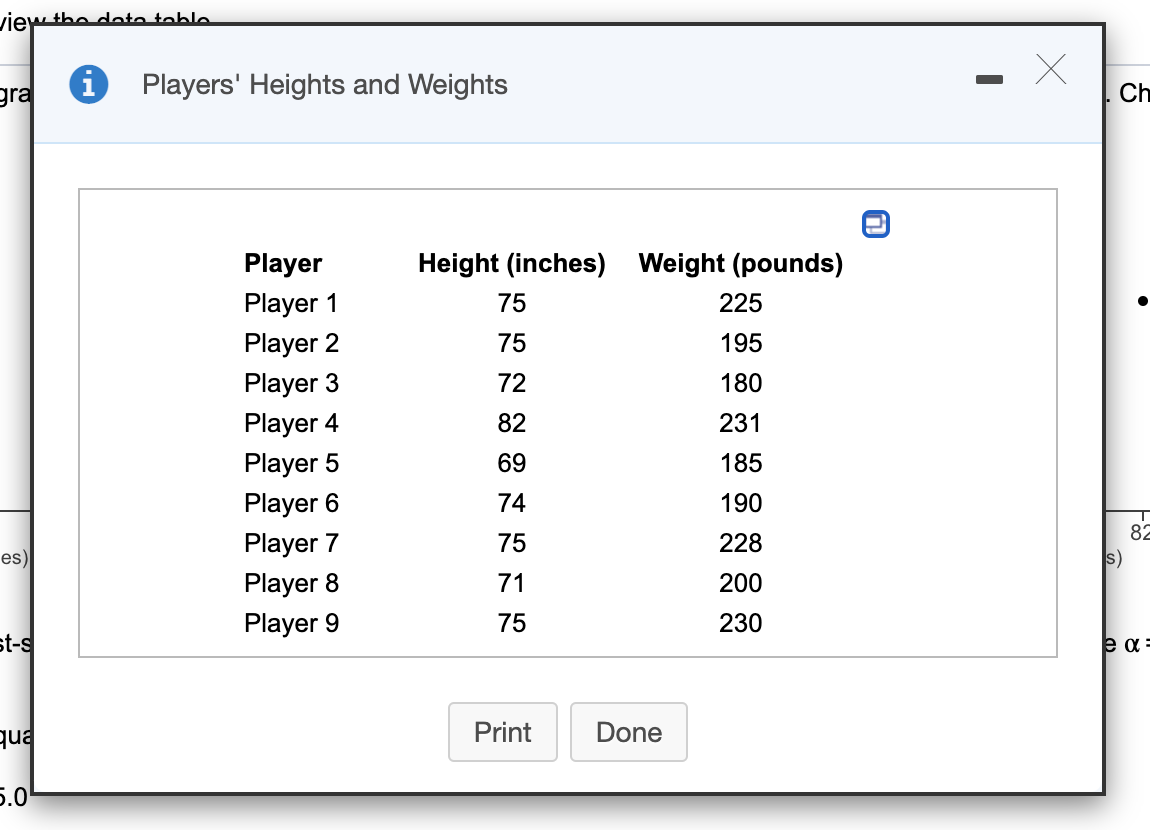
The data in the accompanying table represent the heights and weights of a random sample of professional baseball players. Complete parts (a) through (c) below. a Click the icon to View the data table. (a) Draw a scatter diagram of the data, treating height as the explanatory variable and weight as the response variable. Choose the correct graph below. O A. O B. O c. 240 240 240 'm' 73 '0? 'D 'U U s g g a 210 g 210 g 210 E E E E1 2' .9 d) 0 d) a a a 180 180 180 70 76 82 7O 76 82 7O 76 82 Height (inches) Height (inches) Height (inches) 0 Players' Heights and Weights Player Height (inches) Weight (pounds) Player 1 75 225 Player 2 75 195 Player 3 72 180 Player 4 82 231 Player 5 69 185 Player 6 74 190 Player 7 75 228 Player 8 71 200 Player 9 75 230 Print Done \fTest whether there is a linear relation between height and weight at the o: = 0.05 level of signicance. State the null and alternative hypotheses. Choose the correct answer below. 0 A. H0: o = 0 H1 : o #5 0 QB. H0:|31=0 H1:|31#0 O C. H0:B0=0 H1550>0 O D. H0.B1=0 "'13I31>'0 Determine the Pvalue for this hypothesis test. P-value = D (Round to three decimal places as needed.) State the appropriate conclusion at the a = 0.05 level of significance. Choose the correct answer below. O A. Do not reject Ho. There is not sufficient evidence to conclude that a linear relation exists between the height and weight of baseball players. O B. Do not reject Ho. There is sufficient evidence to conclude that a linear relation exists between the height and weight of baseball players. O C. Reject Ho. There is not sufficient evidence to conclude that a linear relation exists between the height and weight of baseball players. O D. Reject Ho. There is sufficient evidence to conclude that a linear relation exists between the height and weight of baseball players. (c) Remove the values listed for Player 4 from the data table. Test whether there is a linear relation between height and weight. Do you think that Player 4 is influential? Determine the P-value for this hypothesis test. P-value = (Round to three decimal places as needed.)State the appropriate conclusion at the a = 0.05 level of significance. Choose the correct answer below. O A. Do not reject Ho. There is not sufficient evidence to conclude that a linear relation exists between the height and weight of baseball players. O B. Reject Ho. There is sufficient evidence to conclude that a linear relation exists between the height and weight of baseball players. O C. Do not reject Ho. There is sufficient evidence to conclude that a linear relation exists between the height and weight of baseball players. O D. Reject Ho. There is not sufficient evidence to conclude that a linear relation exists between the height and weight of baseball players. Do you think that Player 4 is influential? O Yes O No