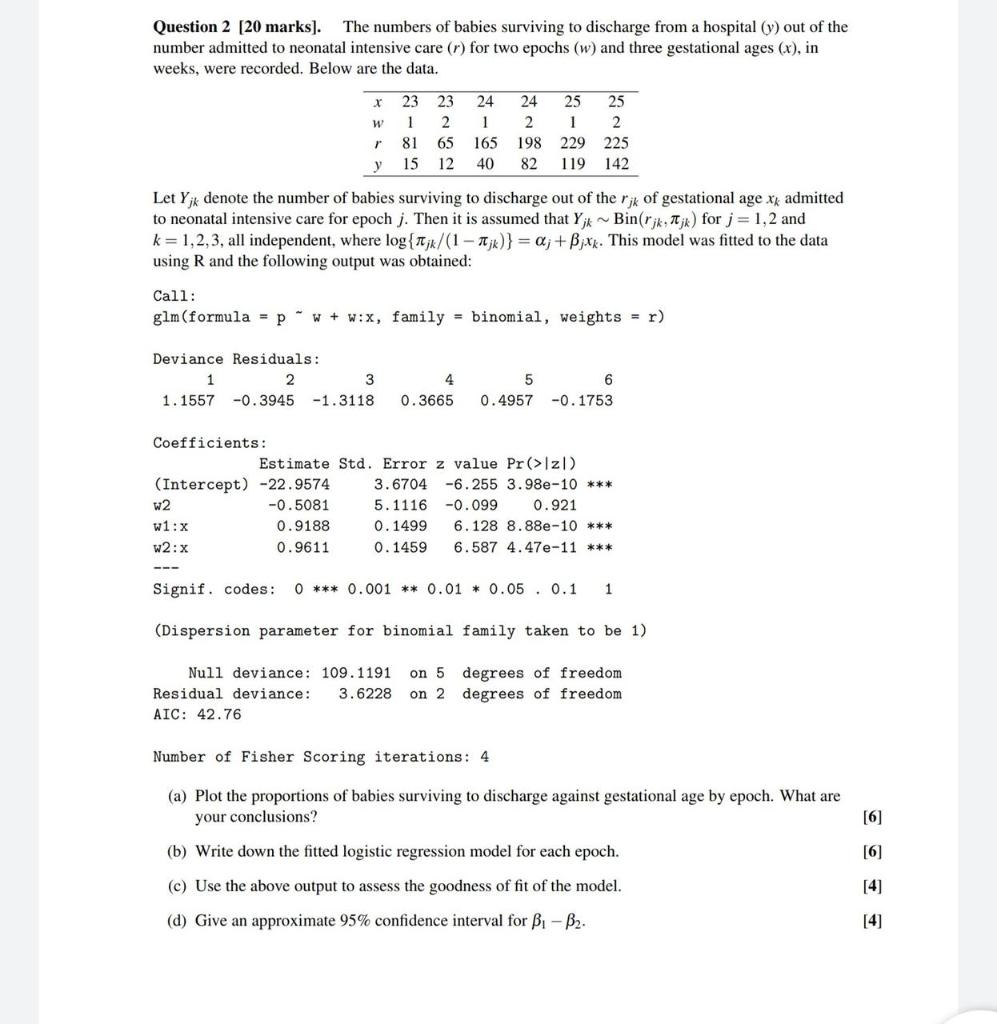
Question 2 [20 marks). The numbers of babies surviving to discharge from a hospital (y) out of the number admitted to neonatal intensive care (r) for two epochs (w) and three gestational ages (x), in weeks, were recorded. Below are the data. X 23 1 81 15 23 2 65 12 24 1 165 40 24 2 198 82 25 1 229 119 25 2 225 142 r y Let Yjx denote the number of babies surviving to discharge out of the rjk of gestational age xx admitted to neonatal intensive care for epoch j. Then it is assumed that Yjx Bin(rik. Ajk) for j = 1,2 and k=1,2,3, all independent, where log{rk/(1 - Aik)} = a;+B;xk. This model was fitted to the data using R and the following output was obtained: Call: glm(formula = pw + w:x, family = binomial, weights = r) Deviance Residuals: 1 2 3 1.1557 -0.3945 -1.3118 4 0.3665 5 0.4957 6 -0.1753 Coefficients: Estimate Std. Error z value Pr(>21) (Intercept) -22.9574 3.6704 -6.255 3.98e-10 *** w2 -0.5081 5. 1116 -0.099 0.921 wi:x 0.9188 0.1499 6.128 8.88e-10 *** 0.9611 0.1459 6.587 4.47e-11 *** w2:x Signif. codes: O *** 0.001 ** 0.01 * 0.05 0.1 1 (Dispersion parameter for binomial family taken to be 1) Null deviance: 109.1191 on 5 degrees of freedom Residual deviance: 3.6228 on 2 degrees of freedom AIC: 42.76 Number of Fisher Scoring iterations: 4 (a) Plot the proportions of babies surviving to discharge against gestational age by epoch. What are your conclusions? [6] [6] (b) Write down the fitted logistic regression model for each epoch. (c) Use the above output to assess the goodness of fit of the model. (d) Give an approximate 95% confidence interval for Bi-B2. Question 2 [20 marks). The numbers of babies surviving to discharge from a hospital (y) out of the number admitted to neonatal intensive care (r) for two epochs (w) and three gestational ages (x), in weeks, were recorded. Below are the data. X 23 1 81 15 23 2 65 12 24 1 165 40 24 2 198 82 25 1 229 119 25 2 225 142 r y Let Yjx denote the number of babies surviving to discharge out of the rjk of gestational age xx admitted to neonatal intensive care for epoch j. Then it is assumed that Yjx Bin(rik. Ajk) for j = 1,2 and k=1,2,3, all independent, where log{rk/(1 - Aik)} = a;+B;xk. This model was fitted to the data using R and the following output was obtained: Call: glm(formula = pw + w:x, family = binomial, weights = r) Deviance Residuals: 1 2 3 1.1557 -0.3945 -1.3118 4 0.3665 5 0.4957 6 -0.1753 Coefficients: Estimate Std. Error z value Pr(>21) (Intercept) -22.9574 3.6704 -6.255 3.98e-10 *** w2 -0.5081 5. 1116 -0.099 0.921 wi:x 0.9188 0.1499 6.128 8.88e-10 *** 0.9611 0.1459 6.587 4.47e-11 *** w2:x Signif. codes: O *** 0.001 ** 0.01 * 0.05 0.1 1 (Dispersion parameter for binomial family taken to be 1) Null deviance: 109.1191 on 5 degrees of freedom Residual deviance: 3.6228 on 2 degrees of freedom AIC: 42.76 Number of Fisher Scoring iterations: 4 (a) Plot the proportions of babies surviving to discharge against gestational age by epoch. What are your conclusions? [6] [6] (b) Write down the fitted logistic regression model for each epoch. (c) Use the above output to assess the goodness of fit of the model. (d) Give an approximate 95% confidence interval for Bi-B2







