Show that the following three problems are polynomially reducible to each other.

(i) Determine, for a given graph G = 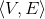and a positive integer m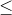 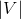, whether G contains a clique of size m or more. (A clique of size k in a graph is its complete subgraph of k vertices.)

(ii) Determine, for a given graph G = 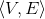and a positive integer m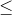 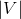, whether there is a vertex cover of size m or less for G. (A vertex cover of size k for a graph G = 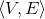is a subset V' 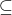V such that 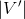= k and, for each edge (u, v) 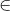E, at least one of u and v belongs to V'.)

(iii) Determine, for a given graph G = 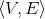and a positive integer m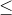 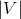,whether G contains an independent set of size m or more. (An independent set of size k for a graph G = 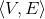is a subset V' 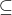V such that 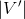= k and for all u, v 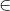V', vertices u and v are not adjacent in G.)