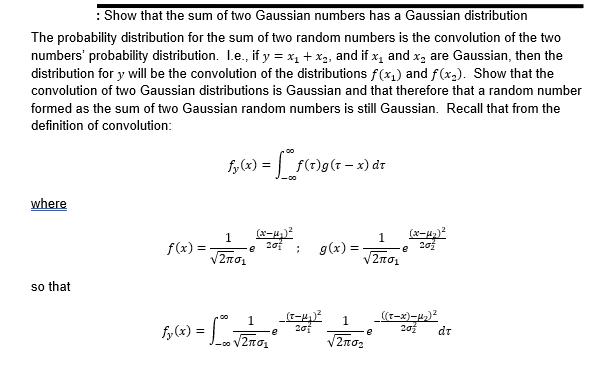
: Show that the sum of two Gaussian numbers has a Gaussian distribution The probability distribution for the sum of two random numbers is the convolution of the two numbers' probability distribution. I.e., if y = x + x, and if x and x are Gaussian, then the distribution for y will be the convolution of the distributions f(x) and f(x). Show that the convolution of two Gaussian distributions is Gaussian and that therefore that a random number formed as the sum of two Gaussian random numbers is still Gaussian. Recall that from the definition of convolution: fy(x) = f(t)g (r - x) dr where so that 1 (x-) f(x) = -no fy(x) = +00 201 ; g(x)= = 1 _(T-H) 201 20 1 20 1 _((T-x)-) 201 20 (x-) 8 20 e dr Hint: The shortcut for performing the convolution is to use the convolution theorem of Fourier analysis. First, take the Fourier transforms of the two distributions, f(x) and g(x), then multiply them together, and then take the inverse transform of that product.