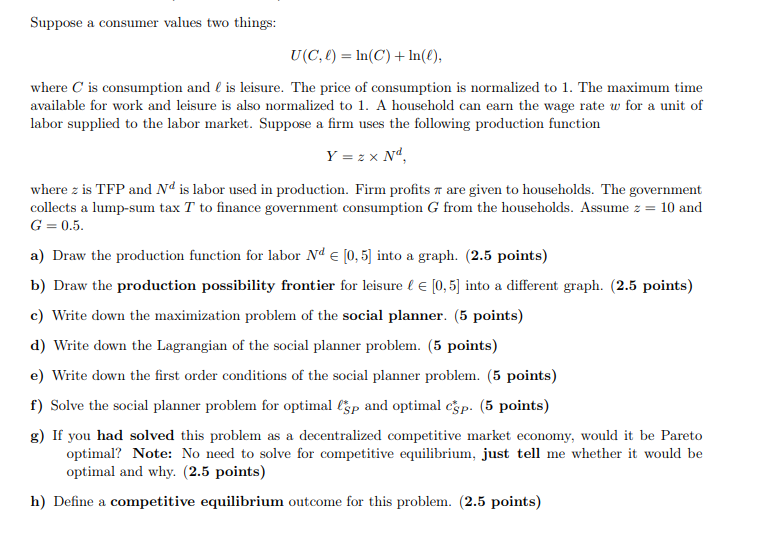
Suppose a consumer values two things: U(C, l)=In(C) + In(l), where C is consumption and is leisure. The price of consumption is normalized to 1. The maximum time available for work and leisure is also normalized to 1. A household can earn the wage rate w for a unit of labor supplied to the labor market. Suppose a firm uses the following production function Y = zx Nd, where z is TFP and Nd is labor used in production. Firm profits are given to households. The government collects a lump-sum tax T to finance government consumption G from the households. Assume z = 10 and G = 0.5. a) Draw the production function for labor Nd [0,5] into a graph. (2.5 points) b) Draw the production possibility frontier for leisure & [0,5] into a different graph. (2.5 points) c) Write down the maximization problem of the social planner. (5 points) d) Write down the Lagrangian of the social planner problem. (5 points) e) Write down the first order conditions of the social planner problem. (5 points) f) Solve the social planner problem for optimal sp and optimal csp. (5 points) g) If you had solved this problem as a decentralized competitive market economy, would it be Pareto optimal? Note: No need to solve for competitive equilibrium, just tell me whether it would be optimal and why. (2.5 points) h) Define a competitive equilibrium outcome for this problem. (2.5 points)