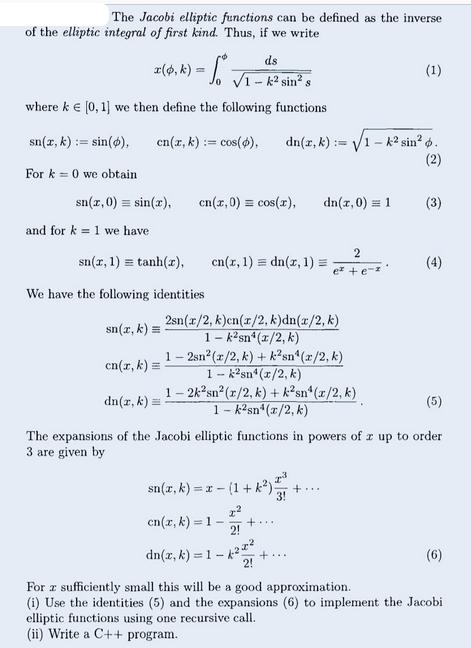
The Jacobi elliptic functions can be defined as the inverse of the elliptic integral of first kind. Thus, if we write ds x(0, k) = 1-k sin s where k = [0,1] we then define the following functions (1) sn(x, k)=sin(), cn(x,k) := cos(0). dn(x, k)=1 k sin. - (2) For k 0 we obtain. sn(x, 0)=sin(x), cn(x, 0) = cos(x), dn(z,0) = 1 (3) and for k 1 we have. 2 sn(2,1) = tanh(r), cn(x, 1) dn(x, 1) = (4) e + e-z We have the following identities sn(x, k) = 2sn(x/2, k)cn(r/2, k)dn(x/2, k) 1- ksn (x/2, k) 1-2sn2(x/2, k) + ksn (x/2, k) cn(x, k) = 1-k2sn(x/2, k) dn(x, k) = 1-2k2sn(r/2, k) + ksn(x/2, k) 1-k2sn(x/2, k) (5) The expansions of the Jacobi elliptic functions in powers of r up to order 3 are given by sn(x, k) = =x-(1+k). +... cn(x, k) 1- +... 2! dn(x, k) = 1-4+... 2! For a sufficiently small this will be a good approximation. (6) (i) Use the identities (5) and the expansions (6) to implement the Jacobi elliptic functions using one recursive call. (ii) Write a C++ program.