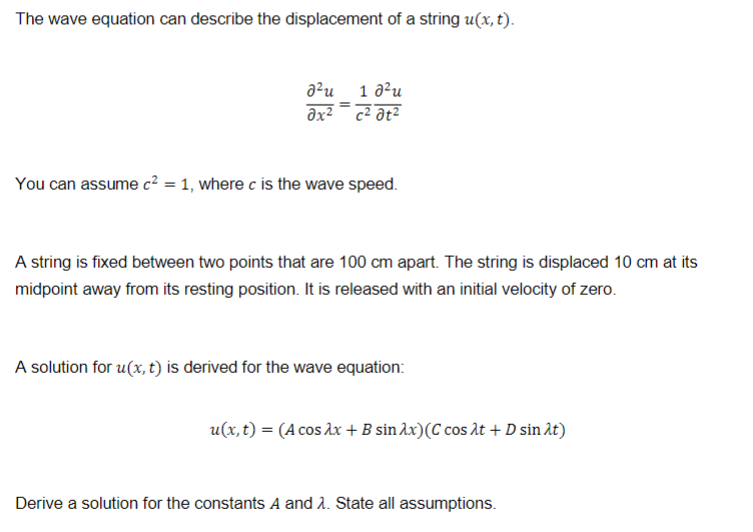
The wave equation can describe the displacement of a string u(x, t). 1 0u 2u x = c t You can assume c = 1, where c is the wave speed. A string is fixed between two points that are 100 cm apart. The string is displaced 10 cm at its midpoint away from its resting position. It is released with an initial velocity of zero. A solution for u(x, t) is derived for the wave equation: u(x, t) = (A cos x +B sin x) (C cost + D sin t) Derive a solution for the constants A and . State all assumptions.