Two economies Rich (R) and Poor (P) are described by the Solow growth model and they share the same Cobb-Douglas production function:

F(K,L)= AK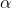 L^1-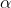

but with different quantities of capital and labour. Economy Rs saving rate is 32% while P's is 10%. The population growth rate of R and P is 1% and 3%, respectively.

Both economies share common technological progress at a rate of 2% annually and depreciation at a rate of 5% annually.

Write down the per worker production function f(k). [2]

Solve the ratio of Rs steady state income per worker to Ps. [Hint: The parameter 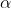will play a role in your answer.] [4]

Supposed the Cobb-Douglas parameter 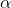takes the conventional value of 1/3 How much higher should income per worker be in economy R than in economy P? [2]

Income per worker in R is actually 16 times higher than income per worker in P. Can you explain this fact by changing the value of the parameter 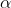? What must it be? Can you think of any way to justify such a value for this parameter? How else might you explain the significant difference in income between economy R and P? [4]