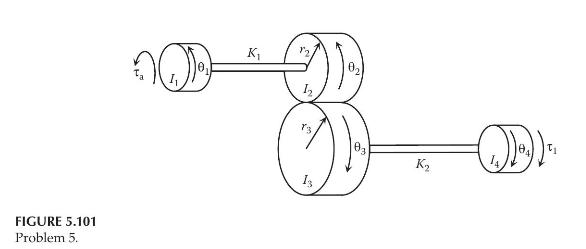
A three-degree-of-freedom gear-train system is shown in Figure 5.101, which consists of four gears of moments of inertia \(I_{1}, I_{2}, I_{3}\), and \(I_{4}\). Gears 2 and 3 are meshed and their radii are \(r_{2}\) and \(r_{3}\), respectively. Gears 1 and 2 are connected by a relatively long shaft, and gears 3 and 4 are connected in the same way. The shafts are assumed to be flexible, and can be approximated by torsional springs. The applied torque and load torque are \(\tau_{\mathrm{a}}\) and \(\tau_{l}\) on gear 1 and gear 4 , respectively. The gears are assumed to be rigid and have no backlash. Derive the differential equations of motion.

Transcribed Image Text:

FIGURE 5.101 Problem 5. K 13 K