We wish to generalize the findings in Exercise 3.8 , and thus consider a stress field of the general form σ_ij = Pf_ij (xk), where P is a loading parameter and the tensor function f_ij specifies only the field distribution. Show that the principal stresses will be a linear form in P, that is, σ_1,2,3 = P_g1,2,3(xk). Next demonstrate that the principal directions will not depend on P.

Data from exercise 3.8

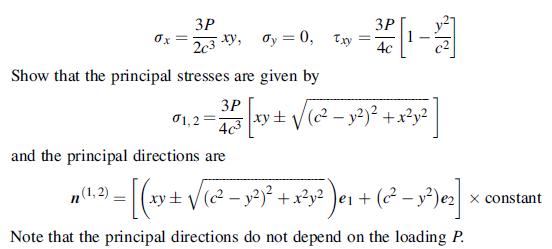
Exercise 8.2 provides the plane stress (see Exercise 3.5) solution for a cantilever beam of unit thickness, with depth 2c, and carrying an end load of P with stresses given by:

Transcribed Image Text:

Ox 3P 2c3-xy, y = 0, Show that the principal stresses are given by 3P 463 01.2= and the principal directions are Txy = [1-3] 3P 4c [xy+ y (c - y) + x ) = [ (xy (c - y) + xy )e + (c - y) e] Note that the principal directions do not depend on the loading P. x constant