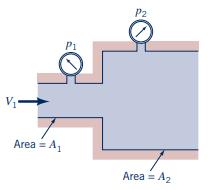
The pressure rise, \(\Delta p=p_{2}-p_{1}\), across the abrupt expansion of Fig. P7.38 through which a liquid is flowing can be expressed as

\[ \Delta p=f\left(A_{1}, A_{2}, ho, V_{1}\right) \]

where \(A_{1}\) and \(A_{2}\) are the upstream and downstream cross-sectional areas, respectively, \(ho\) is the fluid density, and \(V_{1}\) is the upstream velocity. Some experimental data obtained with \(A_{2}=1.25 \mathrm{ft}^{2}\), \(V_{1}=5.00 \mathrm{ft} / \mathrm{s}\), and using water with \(ho=1.94 \mathrm{slugs} / \mathrm{ft}^{3}\) are given in the following table:

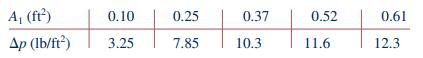
Plot the results of these tests using suitable dimensionless parameters. With the aid of a standard curve fitting program determine a general equation for \(\Delta p\) and use this equation to predict \(\Delta p\) for water flowing through an abrupt expansion with an area ratio \(A_{1} / A_{2}=0.35\) at a velocity \(V_{1}=3.75 \mathrm{ft} / \mathrm{s}\).

Figure P7.38

Transcribed Image Text:

A (ft) 0.10 0.25 0.37 0.52 0.61 Ap (lb/ft) 3.25 7.85 10.3 11.6 12.3