Combine the results of Probs. 10–26 and 10–28 to show that for a two-dimensional slipper-pad bearing, pressure gradient dP/dx is related to gap height

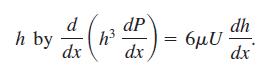
This is the steady, two-dimensional form of the more general Reynolds equation for lubrication (Panton, 2005).

Data from Problem 28

For the slipper-pad bearing of Prob. 10–23, use the continuity equation, appropriate boundary conditions, and the one-dimensional Leibniz theorem to show that

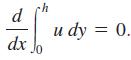
Data from Problem 23

A slipper-pad bearing (Fig. P10–23) is often encountered in lubrication problems. Oil flows between two blocks; the upper one is stationary, and the lower one is moving in this case. The drawing is not to scale; in actuality, h ≪ L. The thin gap between the blocks converges with increasing x. Specifically, gap height h decreases linearly from h₀ at x = 0 to h_L at x = L. Typically, the gap height length scale h₀ is much smaller than the axial length scale L. This problem is more complicated than simple Couette flow between parallel plates because of the changing gap height. In particular, axial velocity component u is a function of both x and y, and pressure P varies nonlinearly from P = P₀ at x = 0 to P = P_L at x = L. (∂P/∂x is not constant). Gravity forces are negligible in this flow field, which we approximate as two-dimensional, steady, and laminar. In fact, since h is so small and oil is so viscous, the creeping flow approximations are used in the analysis of such lubrication problems. Let the characteristic length scale associated with x be L, and let that associated with y be h₀ (x ∼ L and y ∼ h₀). Let u ∼ V. Assuming creeping flow, generate a characteristic scale for pressure difference ΔP = P – P₀ in terms of L, h₀, μ, and V.

FIGURE P10–23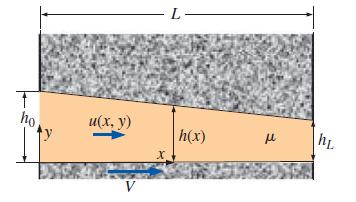

Data from Problem 26

Consider again the slipper-pad bearing of Prob. 10–23.

List appropriate boundary conditions on u. 

Solve the creeping flow approximation of the x-momentum equation to obtain an expression for u as a function of y (and indirectly as a function of x through h and dP/dx, which are functions of x). You may assume that P is not a function of y. Your final expression should be written as u(x, y) = f(y, h, dP/dx, V, and μ). Name the two distinct components of the velocity profile in your result. 

Nondimensionalize your expression for u using these appropriate scales:

Transcribed Image Text:

h by (1 d dx h³ dP dx σμυ dh dx