Consider the case of Maxwell molecules, for which the interparticle force is of the form where K
Question:
Consider the case of Maxwell molecules, for which the interparticle force is of the form
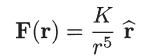
where K is a constant.
(a) Without specifying the form of the distribution functions fα(v) and fβ(v1) for the particles of type α and β, show that the time rate of change of momentum for the particles of type α per unit volume, due to collisions, is given by
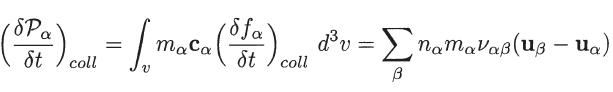
where ναβ is the collision frequency for momentum transfer, given explicity by
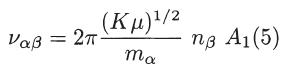
where A1(5) is a dimensionless number (of order unity) defined by (with p = 5 and ℓ = 1)
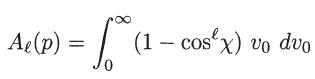
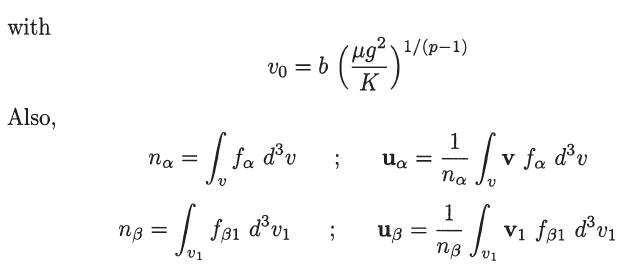
(b) For the same case, show that the time rate of change of energy for the particles of type α per unit volume, due to collisions, is given by
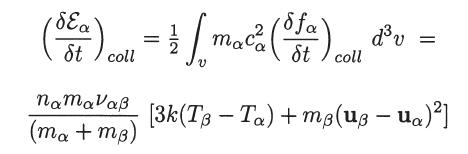
where
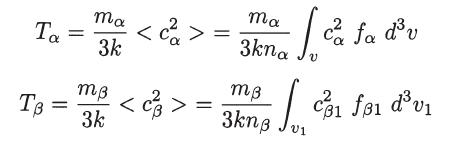
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

Question Posted:





