Finish the proof of Theorem 18.12 (i) and show that every set (A subset mathbb{R}^{n}) is indeed

Question:

Finish the proof of Theorem 18.12 (i) and show that every set $A \subset \mathbb{R}^{n}$ is indeed $\overline{\mathcal{H}}^{0}$-measurable.

Data from theorem 18.12

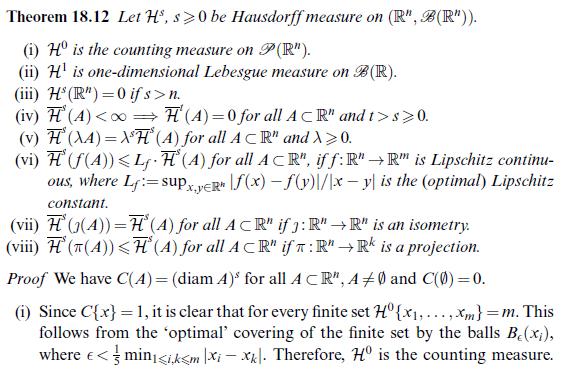
Theorem 18.12 Let Hs, s20 be Hausdorff measure on (R", B(R")). (i) H is the counting measure on P(R"). (ii) H

It is a simple exercise to show that (R") are indeed the H-measurable sets. [] (ii) By Theorem 18.7 (R) C*.

(iv) We know for any E-cover (Si)iEN of A and t>s> 0 that (diam S;)

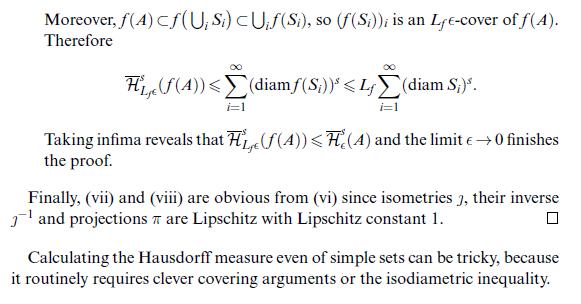
Moreover, f(4) Cf(U; Si) CU,f(Si), so (f(Si)); is an Le-cover of f(A). Therefore He(f(A))

Fantastic news! We've Found the answer you've been seeking!

Step by Step Answer:

Related Book For book-img-for-question

Measures Integrals And Martingales

ISBN: 9781316620243

2nd Edition

Authors: René L. Schilling

See More Books

Question Posted: Jan 25, 2024 01:00 AM

See More Questions

If one attempted to dualize the notion of free module over a ring R (and called the object so defined "co-free") the definition would read: An R-module F is co-free on a set X if there exists a...
Completing a detail of the proof of Theorem 12.5, let G be a finite group of plane isometries. Show that the rotations in G, together with the identity isometry, form a subgroup H of G, and that...
Completing a detail in the proof of Theorem 12.5, let G be a finite group consisting of the identity isometry and rotations about one point P in the plane. Show that G is cyclic, generated by the...
Solve each system using the substitution method. If a system is inconsistent or has dependent equations, say so. -5x + 2y = -2 x + 6y = 26
If the mass of the proton were just a little closer to the mass of the neutron, the following reaction would be possible even at low collision energies: e + p n + v. why would this situation be...
HRH Collection Agency retains a collection fee of 25% of any amounts collected. How much did the agency collect on a bad debt if the agency forwarded $2490 to a client?
Do you have the opportunity to do what you do best every day? 55 45 LO.1
Comparing Cash Flow Streams youve just joined the investment banking firm of Dewey, Cheatum, and Howe. Theyve offered you two different salary arrangements. You can have $90,000 per year for the next...
Problem 9.28 (Break-even Analysis - Low Demand and High Demand) (a) Describe briefly three different types of standard which may be used as the basis for a standard costing system. (b) Two competing...
Let $(X, \mathscr{A}, \mu)$ be a $\sigma$-finite measure space and let $u$ be a further measure. Show that $u \leqslant \mu$ entails that $u=f \mu$ for some (a.e. uniquely determined)...
Show that the outer regularity from Corollary 18.10 coincides with the usual notion, i.e. \[\overline{\mathcal{H}}^{\phi}(A)=\inf \left\{\mathcal{H}^{\phi}(U): U \supset A, U \text { open }ight\},\]...
In Problem, find the particular solution to each differential equation. (x + 1) dy = y 2 dx when x = 0, y = 2
Avery, an unmarried taxpayer, had the following income items: Salary Net income from a rental house 3 7 , 0 5 0 4 , 9 0 0 Avery has a 4 - year - old child who attends a child care center. Assume the...
California Lottery Let A denote the event of placing a $1 straight bet on the California Daily 4 lottery and winning. There are 10,000 different ways that you can select the four digits (with...
"Tamara Wiley glanced in the mirror before leaving her apartment and heading to her 8 a.m. class. She was having a bad hair day, so she had thrown on a scarf. Her quick check in the mirror told her...
Online Friends In a Pew Research Center survey of 1060 teens aged 13 to 17, it was found that 604 (or 57.0%) of those respondents have made new friends online. If the true rate is 50%, there is a...
Dr. Yong has requested that Senture Houston, an office manager at Pain Free Dental Associates, prepare a single journal entry for December 31, 2022. The bank statement for that day shows $9,500....
Air flowing steadily in a nozzle experiences a normal shock at a Mach number of Ma = 2.6. If the pressure and temperature of air are 58 kPa and 270 K, respectively, upstream of the shock, calculate...
Will the prediction interval always be wider than the estimation interval for the same value of the independent variable? Briefly explain.
Explain the meaning of the comparative performance and comparative cost data in Exhibits 18-3 and 18-4. Why does it appear that eliminating sales areas D and E would be profit able?
Most sales forecasting is subject to some error (perhaps 5 to 10 percent). Should we then expect variations in sales performance of 5 to 10 percent above or below quota? If so, how should we treat...
Why is there controversy between the advocates of the full-cost and the contribution-margin approaches to cost analysis?
nformation pertaining to Noskey Corporation s sales revenue follows: November 2 0 2 1 ( Actual ) December 2 0 2 1 ( Budgeted ) January 2 0 2 2 ( Budgeted ) Cash sales $ 1 0 5 , 0 0 0 $ 1 1 5 , 0 0 0...
The management team of Netflix maintains a stable dividend using the Lintner model: Dt+1 = Dt + EPS Target Payout Where Dt (Dt+1) = dividend in the current period t (the next period t + 1) EPSt =...
#1 #2 hapter 50 10 D Werences lav Help Required information [The following information applies to the questions displayed below) Archer Company is a wholesaler of custom-built air-conditioning units...

Finish the proof of Theorem 18.12 (i) and show that every set (A subset mathbb{R}^{n}) is indeed

Question:

Theorem 18.12 Let Hs, s20 be Hausdorff measure on (R", B(R")). (i) H is the counting measure on P(R"). (ii) H is one-dimensional Lebesgue measure on B(R). (iii) H (R")=0 ifs>n. (iv) (4) 0. (vi) H(f(A))

Step by Step Answer:

Data from theorem 185 Fix A C R We have to show tha...View the full answer

Measures Integrals And Martingales

Students also viewed these Business questions