Do NOT copy answers from others or else thumb DOWN!!

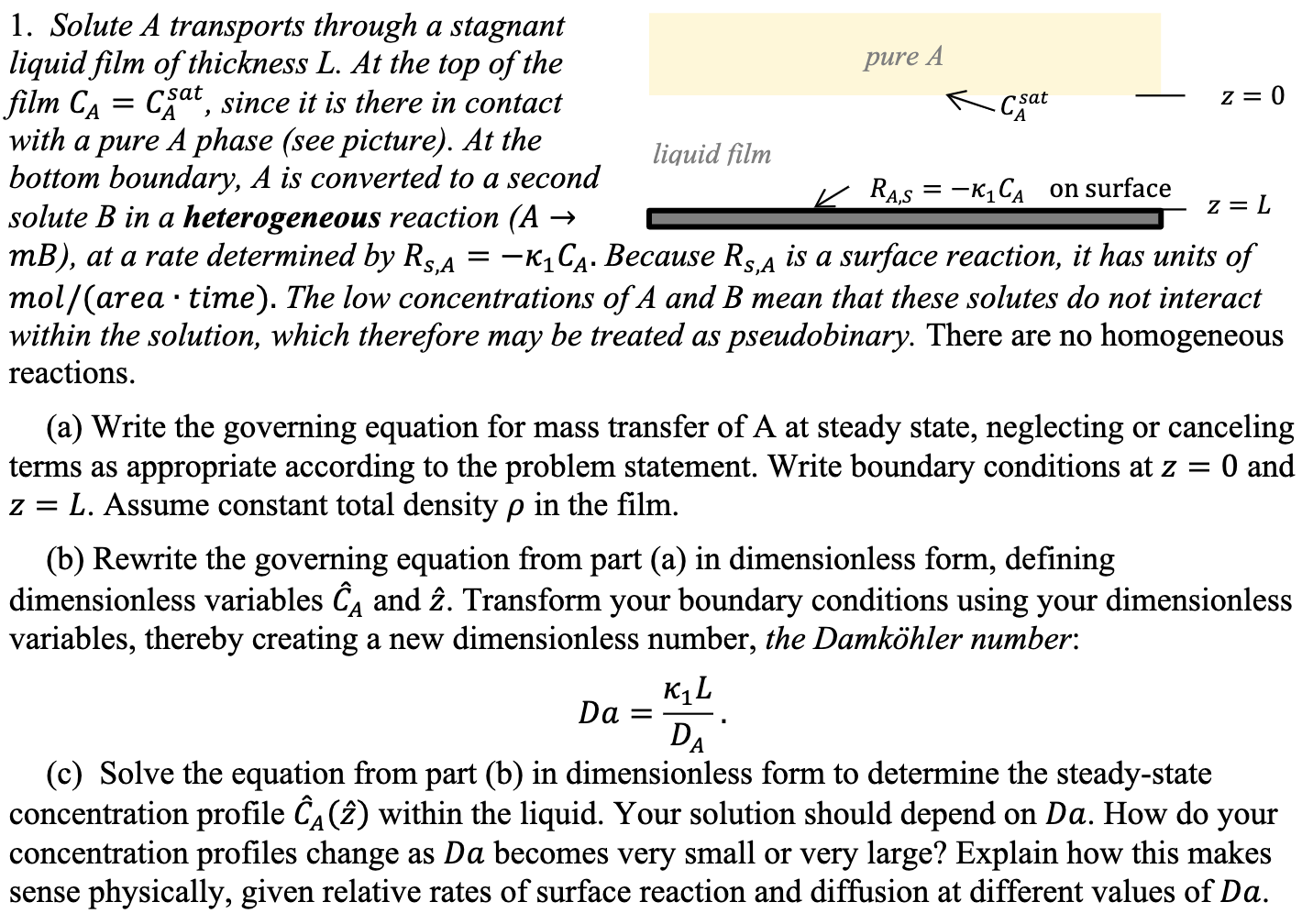
1. Solute A transports through a stagnant liquid film of thickness L. At the top of the film CA=CAsat, since it is there in contact with a pure A phase (see picture). At the bottom boundary, A is converted to a second solute B in a heterogeneous reaction (A mB), at a rate determined by RS,A=1CA. Because RS,A is a surface reaction, it has units of mol/(area -time). The low concentrations of A and B mean that these solutes do not interact within the solution, which therefore may be treated as pseudobinary. There are no homogeneous reactions. (a) Write the governing equation for mass transfer of A at steady state, neglecting or canceling terms as appropriate according to the problem statement. Write boundary conditions at z=0 and z=L. Assume constant total density in the film. (b) Rewrite the governing equation from part (a) in dimensionless form, defining dimensionless variables C^A and z^. Transform your boundary conditions using your dimensionless variables, thereby creating a new dimensionless number, the Damkhler number: Da=DA1L (c) Solve the equation from part (b) in dimensionless form to determine the steady-state concentration profile C^A(z^) within the liquid. Your solution should depend on Da. How do your concentration profiles change as Da becomes very small or very large? Explain how this makes sense physically, given relative rates of surface reaction and diffusion at different values of Da. 1. Solute A transports through a stagnant liquid film of thickness L. At the top of the film CA=CAsat, since it is there in contact with a pure A phase (see picture). At the bottom boundary, A is converted to a second solute B in a heterogeneous reaction (A mB), at a rate determined by RS,A=1CA. Because RS,A is a surface reaction, it has units of mol/(area -time). The low concentrations of A and B mean that these solutes do not interact within the solution, which therefore may be treated as pseudobinary. There are no homogeneous reactions. (a) Write the governing equation for mass transfer of A at steady state, neglecting or canceling terms as appropriate according to the problem statement. Write boundary conditions at z=0 and z=L. Assume constant total density in the film. (b) Rewrite the governing equation from part (a) in dimensionless form, defining dimensionless variables C^A and z^. Transform your boundary conditions using your dimensionless variables, thereby creating a new dimensionless number, the Damkhler number: Da=DA1L (c) Solve the equation from part (b) in dimensionless form to determine the steady-state concentration profile C^A(z^) within the liquid. Your solution should depend on Da. How do your concentration profiles change as Da becomes very small or very large? Explain how this makes sense physically, given relative rates of surface reaction and diffusion at different values of Da