Matlab question

already did part a

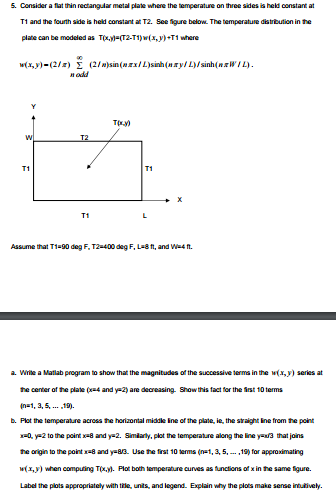
Consider a flat thin rectangular metal plate where the temperature on three sides is held constant at T1 and the fourth side is held constant at T2. See figure below. The temperature distribution in the plate can be modeled as T(x, y) = (T2-T1)w(x, y) + T1 where w(x, y) = (2/pi) sigma^infinity _n odd (2) sin (n pi x/L)sin h(n pi y/L)/sin h(n pi W/L). Assume that T1 = 90 deg F, T2 = 400 deg F, L = 8 ft, and W = 4 ft. Write a Matlab program to show that the magnitudes of the successive terms in the w(x, y) series at the center of the plate (x = 4 and y = 2) are decreasing. Show this fact for the first 10 terms (n = 1, 3, 5, ellipsis, 19). Plot the temperature across the horizontal middle line of the plate, ie, the straight line from the point x = 0, y = 2 to the point x = 8 and y = 2. Similarly, Plot the temperature along the line y = x/3 that joins the origin to the point x = 8 and y = 8/3. Use the first 10 terms (n = 1, 3, 5, ellipsis, 19) for approximating w(x, y) when computing T(x, y). Plot both temperature curves as functions of x in the same figure. Label the plots appropriately with tide, units and legend. Explain why the plots make sense . Consider a flat thin rectangular metal plate where the temperature on three sides is held constant at T1 and the fourth side is held constant at T2. See figure below. The temperature distribution in the plate can be modeled as T(x, y) = (T2-T1)w(x, y) + T1 where w(x, y) = (2/pi) sigma^infinity _n odd (2) sin (n pi x/L)sin h(n pi y/L)/sin h(n pi W/L). Assume that T1 = 90 deg F, T2 = 400 deg F, L = 8 ft, and W = 4 ft. Write a Matlab program to show that the magnitudes of the successive terms in the w(x, y) series at the center of the plate (x = 4 and y = 2) are decreasing. Show this fact for the first 10 terms (n = 1, 3, 5, ellipsis, 19). Plot the temperature across the horizontal middle line of the plate, ie, the straight line from the point x = 0, y = 2 to the point x = 8 and y = 2. Similarly, Plot the temperature along the line y = x/3 that joins the origin to the point x = 8 and y = 8/3. Use the first 10 terms (n = 1, 3, 5, ellipsis, 19) for approximating w(x, y) when computing T(x, y). Plot both temperature curves as functions of x in the same figure. Label the plots appropriately with tide, units and legend. Explain why the plots make sense