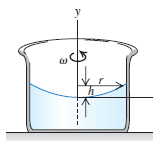
A cylindrical container of an in-compressible liquid with density ρ rotates with constant angular speed ω about its axis of symmetry, which we take to be the y-axis (Fig. P12.86).

(a) Show that the pressure at a given height within the fluid increases in the radial direction (outward from the axis of rotation) according to ˆ‚p/ˆ‚r = ÏÏ‰²r.

(b) Integrate this partial differential equation to find the pressure as a function of distance from the axis of rotation along a horizontal line at y = 0.

(c) Combine the result of part (b) with Eq. (12.5) to show that the surface of the rotating liquid has a parabolic shape; that is, the height of the liquid is given by h(r) = Ï‰²r²/2g. (This technique is used for making parabolic telescope mirrors; liquid glass is rotated and allowed to solidify while rotating.)

Figure P12.86: