a. We have \(150 \mathrm{kmol} / \mathrm{h}\) of a saturated vapor feed at \(1.0 \mathrm{~atm}\) that is \(20 \mathrm{~mol} \%\) methanol and \(80 \mathrm{~mol} \%\) water. This feed is sent to a rectifying column equipped with a total condenser that produces \(20 \mathrm{kmol} / \mathrm{h}\) of distillate that is \(90 \mathrm{~mol} \%\) methanol. CMO is assumed valid, and the column is at \(1.0 \mathrm{~atm}\). VLE data are in Table 2-8. Find B, \(\mathrm{x}_{\text {bot }}\), and the number of equilibrium stages required.

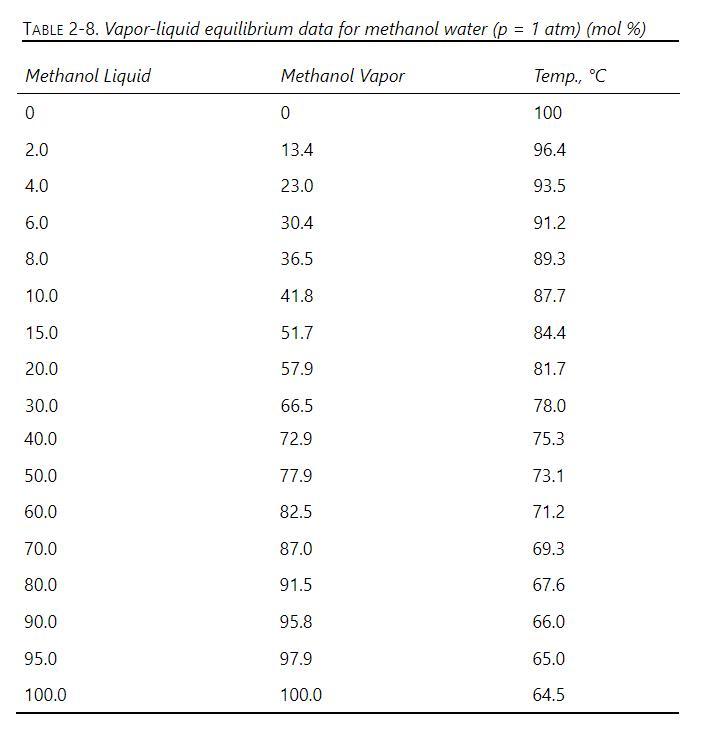
b. A suggested improvement is to take a fraction of the feed, \(\mathrm{f}_{\mathrm{L}}\), condense it to a saturated liquid, and feed it higher in the column than the saturated vapor feed that enters the bottom of the rectifier. For the same problem as in part

a, set \(\mathrm{f}_{\mathrm{L}}=\) 0.3 so that the liquid feed rate is \(\mathrm{F}_{\mathrm{L}}=45.0 \mathrm{kmol} / \mathrm{h}\) and the vapor feed rate is \(\mathrm{F}_{\mathrm{V}}\) \(=105 \mathrm{kmol} / \mathrm{h}\). The two feeds have the same composition ( \(20 \mathrm{kmol} \%\) methanol), but they are not in equilibrium. The column is equipped with a total condenser at the top, and it produces \(20 \mathrm{kmol} / \mathrm{h}\) of distillate that is \(90 \mathrm{~mol} \%\) methanol. CMO is assumed to be valid. Find \(\mathrm{B}, \mathrm{x}_{\text {bot }}\), and the number of equilibrium stages required.

c. Compare the two processes. When do you think the system in part \(\mathrm{b}\) might be more economical?

Transcribed Image Text:

TABLE 2-8. Vapor-liquid equilibrium data for methanol water (p = 1 atm) (mol %) Methanol Liquid Methanol Vapor Temp., C 0 0 100 2.0 13.4 96.4 4.0 23.0 93.5 6.0 30.4 91.2 8.0 36.5 89.3 10.0 41.8 87.7 15.0 51.7 84.4 20.0 57.9 81.7 30.0 66.5 78.0 40.0 72.9 75.3 50.0 77.9 73.1 60.0 82.5 71.2 70.0 87.0 69.3 80.0 91.5 67.6 90.0 95.8 66.0 95.0 97.9 65.0 100.0 100.0 64.5