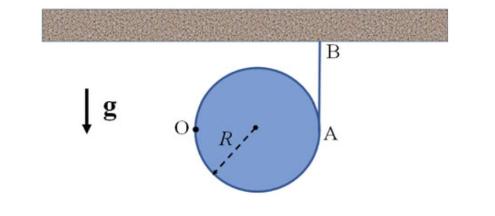
A homogeneous disk, having mass \(M=2.50 \mathrm{~kg}\) and radius \(R=15.0 \mathrm{~cm}\), can rotate, without friction, about a fixed horizontal axis, passing through the point \(\mathrm{O}\) of its circumference and, thanks to the stretched wire AB, is in the equilibrium condition shown in Fig. 12.20. Under these conditions determine:

1. the tension \(\mathbf{T}\) to which the wire \(\mathrm{AB}\) (of negligible mass) is subjected;

2. the force \(\mathbf{Q}_{o}\) due to the constraining reaction present at the point \(\mathrm{O}\).

At a certain instant, the wire is cut and the disk starts rotating about the axis passing through \(\mathrm{O}\). Calculate, at the time when point \(\mathrm{A}\) of the disk comes to rest on the vertical line passing through \(\mathrm{O}\) :

3. the angular speed \(\omega\) with which the disk rotates;

4. the magnitude \(\mathbf{Q}^{\prime}{ }_{o}\) of the force exerted by the constraint at \(\mathrm{O}\).

Fig. 12.20

Transcribed Image Text:

g B A