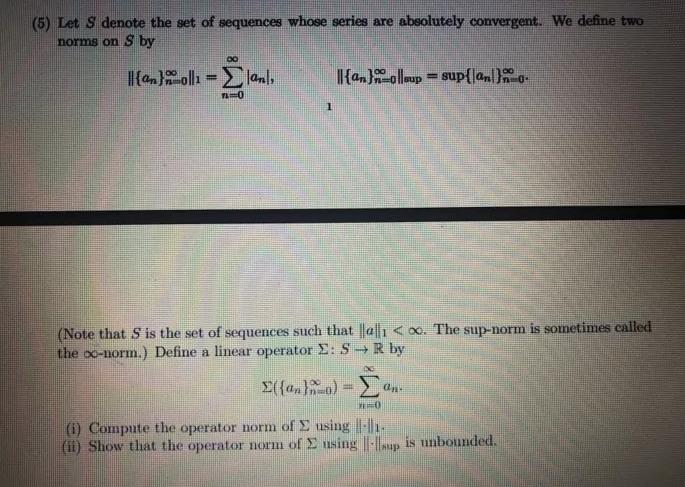
(5) Let S denote the set of sequences whose series are absolutely convergent. We define two norms on S by |||{n}-oll = |an| {anollsup = sup{an) 10 1 (Note that S is the set of sequences such that all1 < oo. The sup-norm is sometimes called the co-norm.) Define a linear operator E: SR by {{cn}50) = -i On =0 (i) Compute the operator norm of using 1. (ii) Show that the operator norm of using up is unbounded.